Question
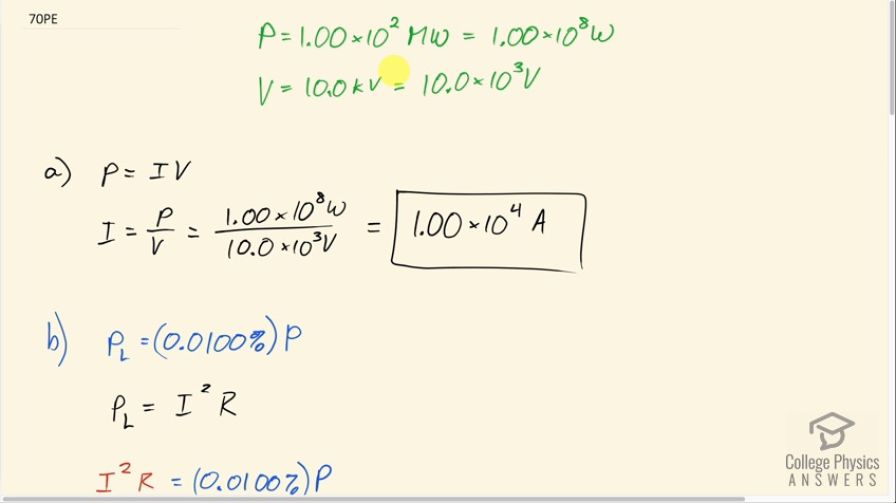
(a) What current is needed to transmit of
power at 10.0 kV? (b) Find the resistance of 1.00 km of wire that would cause a 0.0100% power loss. (c) What is the diameter of a 1.00-km-long copper wire having this resistance? (d) What is unreasonable about these results? (e) Which assumptions are unreasonable, or which premises are inconsistent?
Final Answer
- This diameter is way too large. The cost of copper, and the cost of the sturdy, closely spaced utility poles needed to support such a large mass of copper, would be prohibitive.
- The power loss is too small. Also, such a large power transmission should be transmitted with a higher voltage in order to reduce the current.
Solution video
OpenStax College Physics, Chapter 20, Problem 70 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have some interesting questions about power transmissions through power lines and we are gonna be asked what is the size of a copper wire having 0.0100 percent power loss given that this much power is transmitted at this voltage. So part (a) asks us what the current would be through a transmission line transmitting 1.00 times 10 to the 2 megawatts which is 1.00 times 10 to the 8 watts because the prefix 'mega' means multiplied by 10 to the 6 and 10 to the 6 times 10 to the 2 is 10 to the 8. It's being transmitted at a voltage of 10.0 kilovolts, which is 10.0 times 10 to the 3 volts. We use the P equals IV formula to find the current and we will divide both sides by V to solve for I. So the current is the power divided by the voltage and that works out to 1.00 times 10 to the 4 amps. Part (b) says find the resistance of 1.00 kilometer of wire that would have a power loss of only 0.0100 percent. This power loss then is 0.0100 percent of the total power not necessarily transmitted to the customer but rather, you know, supplied by the source. So 0.0100 percent of the total power supplied by the source is going to be lost and this power loss can also be written as the current in the wire squared times the wire's resistance. So we substitute I squared R in place of P L and then solve for R by dividing both sides by the square of the current. So R then is 0.0100 percent, written as a decimal divided by 100, times by the power transmitted divided by the current squared and that's 0.000100 times 1.00 times 10 to the 8 watts divided by 1.00 times 10 to the 4 amps squared which is 1.00 times 10 to the negative 4 ohms so a very small resistance is required in order to have such a small power loss. Part (c) asks what is the diameter of a 1.00 kilometer long copper wire having this resistance? Well we can find the cross-sectional area of this wire using this formula so the resistance of the wire is the resistivity of copper multiplied by its length divided by its cross-sectional area and we'll solve for A by multiplying both sides by A divided by resistance and then the cross-sectional area is the radius squared times π and then substitute in diameter divided by 2 in place of radius because it's asking us the diameter of the wire and so we have πd squared over 4 is the area and we substitute this for A here and writing that in red here. We are gonna solve for d first by multiplying both sides by 4 over π so this gives us d squared. So d squared equals 4 times resistivity of copper times its length divided by π times its resistance then take the square root of both sides and get d then is 2 times the square root of ρ CuL over πR and this 2 comes from taking the square root of the factor 4 and then writing it outside the radical. So we have 2 times square root of resistivity of copper, which we looked up in table [20.1], multiplied by the length—written in meters— 1.00 kilometer times a 1000 meters per kilometer divided by π times the resistance we found in part (b)— 1.00 times 10 to the minus 4 ohms— this works out to 46.8 centimeters. This diameter is very large for a wire and the cost of so much copper and the cost of the sturdy, closely spaced utility poles needed to support so much mass would be prohibitive. And the reason that this is unreasonable is that the power loss expected of 0.0100 percent is too small and also such a large amount of power— 1.00 times 10 to the 8 watts— should be transmitted with a much higher voltage and a higher voltage would result in a smaller current as you can see from this formula; as the denominator goes up, the quotient would be reduced, in other words, the current would go down with an increase in voltage. And that's why long-distance power lines are very high voltage to reduce the current through them and therefore the power lost in them.
