Question
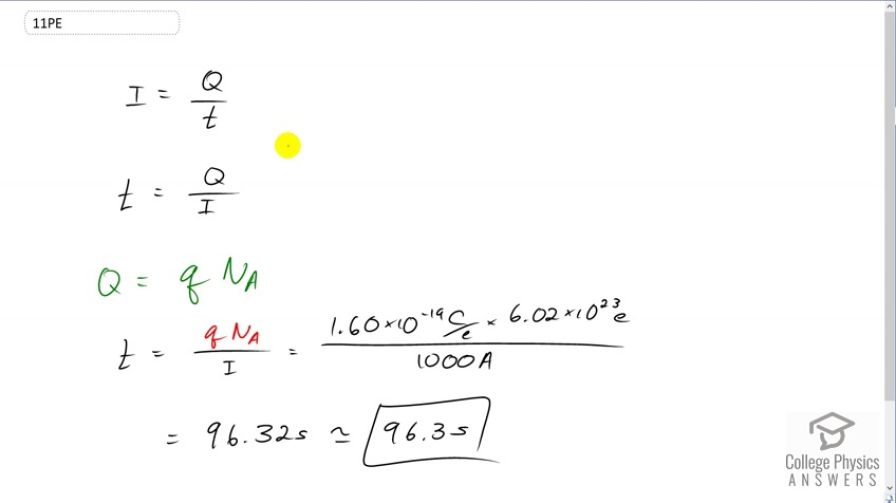
The batteries of a submerged non-nuclear submarine supply 1000 A at full speed ahead. How long does it take to move Avogadro's number ( ) of electrons at this rate?
Final Answer
Solution video
OpenStax College Physics, Chapter 20, Problem 11 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're asked to figure out how long it takes for the batteries in this submarine to supply an Avogadro's number of electrons. Well, let's rearrange this formula for current and solve it for t. Multiply both sides by t over I and the I's cancel on the left isolating t there, on the right hand side the t's cancel leaving us with Q over I. Then we need to figure out what Q is based on the information we're given. We're told that the charge will be -- that the charge on this many electrons, this is Avogadro's number, and we're multiplying that by Q, which is the elementary charge, it's the number of coulombs per electron. So we substitute that in for Q and so we have 1.6 times ten to the minus nineteen coulombs per electron, and then we're multiplying that by 6.02 times ten to the twenty-three electrons. The electron number there cancels, and dividing that by 1000 amps and we get 96.3 seconds is the amount of time it takes for the batteries to discharge 6.02 times ten to the twenty-three electrons.
