OpenStax College Physics for AP® Courses, Chapter 3, Problem 60 (Problems & Exercises)

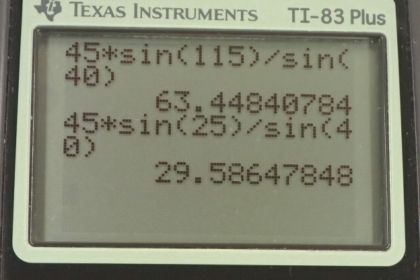
This is College Physics Answers with Shaun Dychko. In this video we’re working on question 60, which is a good challenge question on adding relative velocities. So we're told that an airplane is flying in a jet stream; which means wind, that's blowing 45 meters per second in a direction, 20 degrees south of east. So lets begin by writing down this known, this information that we know. And so we write the velocity of the wind with respect to the ground. That's what the w and the g stand for; wind and ground, is 45 meters per second, 20 degrees south of east. And here are the cardinal directions, and so that's going to be a vector in this direction like this. And this angle here will be 20 degrees. That's 20 degrees south of east. And then the next thing that we know is, and the airplane when it says it, it’s referring to the airplane. Its direction of motion relative to the earth is 45 degrees south of west. So we have another vector, velocity of the airplane with respect to the ground, is some unknown speed. We don't know what that is yet. But we know that it's 45 degrees south of west. So it's going this direction, and this is 45 degrees here. And then we also know about the direction of the airplane with respect to the wind. So, it's direction relative to the wind is five degrees south of west. Alright, so we’ll write down that piece of information as well. So the velocity of the airplane with respect to the wind is some unknown speed, five degrees south of west, so like this. Now when you're, oh, so and then what’s the question? Well, yes that's the important part isn’t it. The question is what is the airplane’s speed compared to the wind? So I'm using, I'm not reading these words verbatim. I’m translating them into the words that I'm using in my solution. So what’s the airplane’s speed compared to the wind? And what is the airplane’s speed compared to the ground? So, when you're adding relative velocities, always write two subscripts; the first subscript says what’s moving. So this is the airplane, so this is the thing that’s moving, is indicated by the first subscript. And the second subscript indicates what the velocity is compared to. So this is the velocity of the airplane relative to the wind. And that's the question we want to answer. And it'll equal the velocity of the airplane with respect to the ground, plus the velocity of the ground with respect to the wind. And you should be, maybe if you haven't seen this sort of technique before, you should be scratching your head when you see that. Because it's a bit strange to think about the ground. What’s the velocity of the ground with respect to the wind? And there's a good reason why I write it this way though. When you’re adding relative velocities, if you have the inner subscripts the same, they cancel. And what you're left with is a velocity of the outer subscripts. So you can be left with the a, and you'll be left with the w. And it'll be velocity of the a with respect to the w, so velocity of the airplane with respect to the wind. Which is what we wanna find here. And to make you believe that in case this is the first time you've seen that: Suppose you have a person running on a train car. And this person has some you know, velocity, five meters per second. But this is the velocity of the person with respect to the train, and then the train itself also has some other velocity. I suppose this train vector should be longer than the person’s vector. Because the train is likely going faster than the person that is running. Velocity of the train with respect to the ground is, say 15 meters per second. And you wanted to know what the velocity of the person with the respect to the ground was. Well, clearly since they're both going the same direction, you're going to just add these two factors together, and the answer will be 20. But I want you to see that that answer comes about using this technique. So we want to know what the velocity of the person with respect to the ground is; so we better add the velocity of the person with respect to the train, plus velocity of the train with respect to the ground. Notice that the inner subscripts are the same, and so they cancel. And we're left with velocity of the person with respect to the ground. And this always works out when you arrange your subscripts this way. So I did not want the velocity of the wind with respect to the ground, even though we're given that. Because then the inner subscripts would be a g and w, and that would be bad. Instead, we want to have the inner subscripts the same; both g and now we can answer our question. So, now, what is the velocity of the ground with respect to the wind? Well it’s going to be the opposite of the velocity of the wind with respect to the ground. When you wanna switch these subscripts around, you just put a negative sign in front of it. So if we have the velocity of the wind with respect to the ground, given to us; 20 degrees south of east, 45 meters per second. The opposite of that is going to be this vector. Which is going 20 degrees north of west, 45 meters per second. So there we go. That's the velocity of the ground with respect to the wind, and that's what we're going to use in this equation here. Now, we're gonna add the two vectors together; the velocity of the air with respect to the ground, plus the velocity of the ground with respect to the wind. And what we'll be left with is the velocity of the air with respect to the wind. So this resultant vector here is going to be what we want to find. Now there's a bunch of angles that we need to plug into this picture in order to solve the triangle. So first of all we're told that the velocity of the airplane with respect to the ground is 45 degrees. And that is south of west. So that's where this number comes from. And we're told that the velocity of the airplane with respect to the wind. Velocity of the airplane with respect to the wind is some unknown magnitude. But with this direction is five degrees south of west. So that means this number is given to us, five degrees south of west. And that means we can figure out this tiny angle inside the triangle, it’s going to be 45 degrees, minus this five degrees and that's where this 40 comes from. And this angle here is 45, because we have interior opposite angles, where this is the transversal between two parallel lines; these dotted lines that are horizontal. And with this being 45 here, that means this is 45 as well. And that's useful because we know given that 45 and given this 20 degrees here, this is the direction of the ground with respect of the wind. This 45 plus this 20 plus this unknown angle theta here, has to add up to a supplementary angle or 180 in other words. So that makes this a hundred and, hang on. Trying to find this angle theta and it's going to be 180, the total straight line angle, minus 45 here, minus 20, leaving us with 115 degrees as the angle theta. So we know what theta is. We know this angle is 40 and we can also figure out this angle alpha. Now, maybe I can draw this in a separate spot here. We have this velocity of the airplane with respect to wind going like that. We have this velocity of the ground with respect to the wind going like this, and we can draw a horizontal here. And this angle here will be an interior opposite angle to this one. And that’s going to be five degrees. And then this angle here will be interior opposite angles compared to this one here, which is going to be 20. And so this total angle is 25 degrees in here. Now, and we know the length of the side. So because we know all the angles and the length of one side, that means we can solve the triangle. We can answer any other question about the triangle that we want. So first we're going to figure out the magnitude of the velocity of the airplane with respect to the wind. And we're going to use the sine law to do that. And the sine law; imagine a triangle where you have sides labeled small a, small b, and small c. The angles opposite to each of those sides would be their capital counterparts. So this is the angle opposite little a, so that makes it capital A. This is angle opposite little b, so that makes it capital B. And this is angle across from little c, which makes it capital C. And the sine law says; if you have a side divided by the sine of it's opposite angle, that's going to equal any other side length divided by the sine of it's opposite angle. So we have v a w is like side c. And so we’re gonna divide it by sine of the angle opposite to it. Which will be theta in our case. And that's why you see a sine of 115 as a factor here. Because I multiply both sides by sine c. So these cancel. Leaving us with c equals little b, times by sine capital c divided by sine capital b. So what is this side length b? It is this 45 meters per second that we know; the velocity of the ground with respect to the wind. And we’re multiplying that by sine of the angle opposite the unknown side, which is theta, 115 degrees. And divide that by the sine of the angle opposite to the known side. And so that's this angle here. This angle is opposite our known side, and so we’re dividing by sine of 40. And this works out to 63.4 meters per second. And I put these absolute value bars here just to remind us that this is the magnitude of that velocity. It’s not the velocity. So this is the speed in other words. And that's the answer to part A. 63.4 meters per second, the speed of the airplane with respect to the wind. Then the question also asks us in part B; What does it say? It says, what is the airplane speed relative to the ground? Well, that is this vector here. And so we’ll use the same sine law technique and figure out this side instead of that one. And so the speed of the airplane with respect to the ground, divided by the sine of it's opposite angle. Which in this picture is alpha. Is going to equal the velocity of the ground with respect to the wind, divided by the sine of that angle opposite it. Which is 40 degrees. And so we multiply both sides by sine alpha. And then we get the speed of the airplane with respect to the ground, is going to be 45 meters per second, multiplied by sine 25, divided by sine of 40. 25 being this angle alpha opposite the velocity of the airplane with respect to the ground. And this works out to 29.6 meters per second.