Question
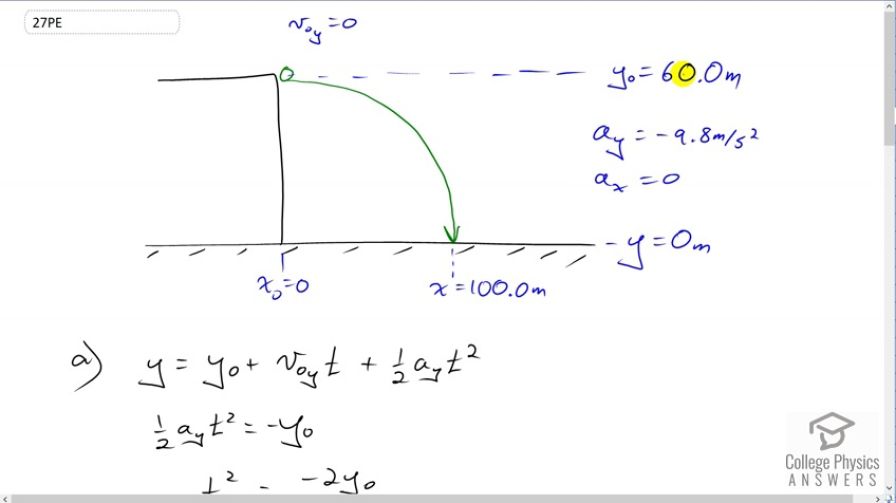
A ball is thrown horizontally from the top of a 60.0-m building and lands 100.0 m from the base of the building. Ignore air resistance. (a) How long is the ball in the air? (b) What must have been the initial horizontal component of the velocity? (c) What is the vertical component of the velocity just before the ball hits the ground? (d) What is the velocity (including both the horizontal and vertical components) of the ball just before it hits the ground?
Final Answer
a)
b)
c)
d)
Solution video
OpenStax College Physics for AP® Courses, Chapter 3, Problem 27 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This ball is thrown horizontally from the top of a building. The building we'll say is 60 meters high, so we'll say that y naught equals 60. The initial y position is 60 and we'll take the ground to be y equals zero. There is no acceleration horizontally but there is gravity and that's going to affect the vertical direction and so the vertical acceleration is negative 9.8 meters per second squared. Initially there is no vertical velocity at all because it says the ball is thrown horizontally. So there is some v subscript x naught but we don't know what that is. That's one of the questions is to find it. So part A asks us for how long is the ball in the air. So we can use this formula to figure that out, the final y position is the initial y position plus the initial y velocity times time, plus one half times the vertical acceleration times time squared. This term is zero because there is no initial vertical velocity and this term here is also zero because the final position is zero. So we can subtract y naught from both sides, then switch the sides around and we get one half ayt squared equals negative y naught. Then, our job here by the way is to solve for t because the question is asking for how long is the ball in the air. So we will isolate t by multiplying both sides by 2 over ay and then we end up with this line t squared equals negative two times the initial y position, divided by the vertical acceleration. Then take the square root of both sides and mathematically speaking we should put a plus or minus here, but physically speaking, we know that the time needs to be positive, there's no physical interpretation for a negative time here. So we'll just take the positive square root. So time is the positive square root of negative two y naught over ay so that's the square root of negative two times 60 divided by negative 9.8 meters per second squared, giving us 3.50 seconds in the air. The next question is asking how far from the base of the building will the ball land. So what is x in other words or, wait a second, that's not the question. No, v naught, yeah, we're given x actually. It's 100 meters. We're told that the ball lands 100 meters from the base of the building. So, v naught x is the question here. Well, we can use this formula because the only unknown is v naught x in here because we know t and we figured it out before in part A and we know what x naught is, it's zero because it starts at position zero. So this is v naught x times the square root of negative two y naught over ay. I could have just written the number 3.5 here but I'm just trying not to use the result of previous parts of the question in subsequent parts and trying to just come up with formulas that use the data that we've been originally given in the question. So I wrote square root negative two y naught over ay as the substitution for t. Then we will solve for v naught x by multiplying both sides by ay over negative two y naught square rooted. Then the same on the other side, and then we end up with this line here after you switch the sides around. You have the horizontal initial velocity which you know is the horizontal velocity in general because it's never changing since there's no horizontal acceleration at all. It equals the square root of ay over negative two y naught, all times the final x position x. So that's square root of negative 9.8 meters per second squared over negative two times 60 meters, all times 100 meters, giving us 28.6 meters per second as the initial horizontal velocity. Then part C is asking for the final y component of its velocity and this formula here will be helpful because we know the initial y component of its velocity is zero because the ball is thrown horizontally so there's no component in the y direction. We know the initial y position is 60 meters. We know the final y position is zero and so this is the formula containing only one unknown which is the thing we want to find. So we'll get rid of this term and then take the square root of both sides and we get vy is negative. Then we put the negative there because we know the ball is going downwards and so the y component of its velocity is negative, times the square root of two times vertical acceleration times the final position minus the initial position. So that's negative two times -- sorry, negative square root of two times negative 9.8, times zero minus 60. That gives negative 34.3 meters per second. Now, putting the x and y components together, we can figure out the final velocity just as it's hitting the ground. So the magnitude of its final velocity will be the square root of the sum of the squares of the components of the velocity. So that's square root of negative 34.29 meters per second squared, and notice I've written more digits here in this working than I had in my final answer for the y component of velocity. That's a good best practice because normally you want to avoid rounding numbers that you use in your calculations. If we put in negative 34.3 here, we will get a slightly different answer actually. Probably we get I think 44.7 and that 44.7 would be called an intermediate rounding error because using a rounded number in the calculation introduces its own error. So we will use un-rounded numbers as much as possible. Then plus the horizontal component of its velocity 28.5774 meters per second squared and square rooting that sum of the squares gives 44.6 meters per second. The direction is going to be the inverse tangent of the y component of velocity divided by the x component of the velocity and we're ignoring the negative signs. So that's why I have these absolute value symbol here, these vertical bars. So that is just to say that you take the inverse tangent of 34.2929 divided by 28.5774 giving us 50.2 degrees. Because the velocity at the end here is down to the right and we've taken the inverse tangent of its y component divided by its x component. The angle that we found is this one. So this angle is below the positive x axis and so our final answer for velocity just before the ball hits the ground is 44.6 meters per second, 50.2 degrees below the positive x axis.

