Question
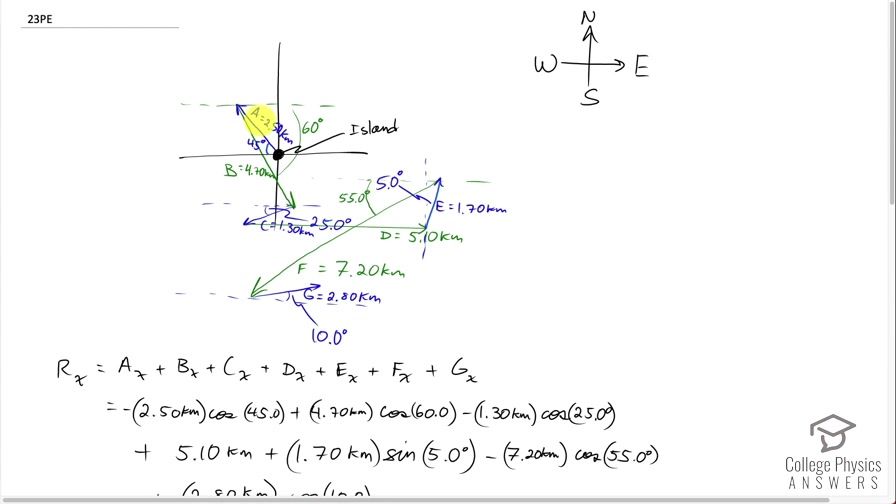
In an attempt to escape his island, Gilligan builds a raft and sets to sea. The wind shifts a great deal during the day, and he is blown along the following straight lines: 2.50 km north of west; then 4.70 km south of east; then 1.30 km south of west; then 5.10 km straight east; then 1.70 km east of north; then 7.20 km south of west; and finally 2.80 km north of east. What is his final position relative to the island?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 3, Problem 23 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
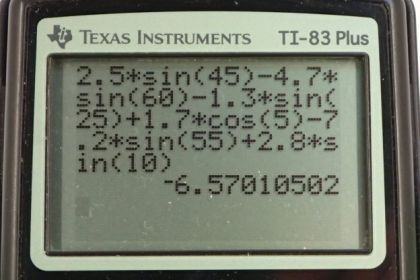
This is College Physics Answers with Shaun Dychko. Gilligan's raft is being blown in many different directions after he departs from this island, he goes along seven different vectors here and our job is to figure out what is Gilligan's final position? So first he travels 2.50 kilometers 45.0 degrees north of west, which is this blue vector, and then 4.70 kilometers 60.0 degrees south of east, which is this vector B, and then 25.0 degrees south of west, which is this vector C— 1.30 kilometers— then 5.10 kilometers directly east and 1.70 kilometers 5.0 degrees east of north and then 7.20 kilometers 55.0 degrees south of west and then 2.80 kilometers finally 10 degrees north of east. So the final position will be the resultant of all these vectors added together and I have placed them head to tail and so the resultant will be from the origin to the head of the last vector so this is the resultant position of the raft and we have to figure out what that is... what is that vector... its length and its direction. So there's two tricky things with this problem: one is that there are so many vectors and so many numbers involved that it's easy to make careless mistakes with copying numbers and so on but another issue is that this vector E is given with an angle compared to a vertical axis and that means that trigonometric function that we use for finding the components of E will be the opposite function that we are accustomed to for every other vector; more conventionally, angles are given with respect to a horizontal axis in which case you use cosine for finding an x component and sin for using a vertical component but in the case of E, the way it's drawn, the horizontal component will be... I am just going to redraw E over here so we can see it more easily. Suppose this is vector E, its horizontal component will be this— this is E x— and then its vertical component will be here—this is E y— and the angle that we are given is this 5.0 degrees in here and so the horizontal component is the opposite leg of this triangle and so we multiply E by sin of the angle 5.0 degrees to find the x component of it so we will get to that in a second but that's just something to be mindful of... the x component for every other vector will be using cosine. Okay! So let's get to work... we have to be very methodical here because there are just so many vectors involved. We need to find what the x component and the y component of the resultant is. So the resultant x component will be this vector here and its y component will be this thing here and the x component of the resultant will be the sum of the x components of all the other vectors that we are adding together. So its the x component of vector A, x component of vector B, C, D, E, F and G. So taking our coordinate system to be positive to the right and positive upwards, which is conventional although not rigidly necessary but we will just follow that usual convention that means the x component of vector A is negative because it's to the left and the x component is this little bit that goes here— that's the x component of vector A— or I have could have drawn it down here so that it more clearly is the adjacent leg of this triangle in yellow here. Okay! So it has a length of 2.50 kilometers—it's negative because it's to the left— and we are going to multiply it by cosine of 45.0 degrees to get the adjacent leg of this triangle and because the adjacent leg is the x component here. Then add to that the x component of vector B, which is to the right and so it's positive multiply 4.70 kilometers by cosine of 60.0 degrees so now we are focusing on this triangle here and it's this little bit here that we are looking for this is B x—the x component of vector B— and that's 4.70 times cosine of 60.0— it's the adjacent leg of this right triangle in yellow. Then we have vector C going somewhat to the left and so it has a negative x component and it's 1.30 kilometers times cosine of 25.0 degrees then vector D is entirely in the x direction so there's no need for a trigonometric function although you could multiply by cosine of 0 if you like and that's got to be 5.10 kilometers to the right—positive— and then we have 1.70 kilometers times sin of 5.0 degrees and we discussed how this is the opposite leg of this triangle— we are looking for the opposite leg of this triangle to find the x component and so that's why we are using sin— and then minus 7.20 kilometers times cosine of 55.0 degrees to get the x component of vector F and so I will outline the vector F triangle in yellow here... let's make it a different color... let's make it light blue so it's this triangle we are concerned with now... and that's 7.20 kilometers times cosine of 55.0 degrees— minus because it's to the left— and then 2.80 kilometers times cosine of 10.0 degrees gives us the adjacent leg of this triangle and that's positive because that component is to the right and so that's 2.80 kilometers times cosine of 10.0. This all works out to 3.2799 kilometers that is the x component of the resultant. Okay! Then we need to think about the y component and pretty much the same process but just thinking about the vertical component. So we have 2.50 kilometers times sin of 45.0 to get this vertical component of vector A and then minus 4.70 kilometers times sin of 60.0 because this vector B is downwards and to the right and so while its x component is positive, its y component is negative because it's going down and so that's the opposite leg of this triangle here and so we are using the sin of 60.0 multiplied by the hypotenuse that's minus 4.70 times sin of 60.0 and then minus 1.30 kilometers times sin 25.0— we are looking for the opposite leg of this triangle now and that's downwards so that's negative as well. Vector D has no y component so we can skip that one. Vector E has a vertical component, which is the adjacent leg of this triangle so we use cosine of 5.0 degrees times 1.70 kilometers (sorry... which one was that... that's this one here) and then vector F is somewhat downwards and so it has a negative y component— that's 7.20 kilometers times sin of 55.0 degrees here's the angle... here's the triangle. And then lastly, we have this little bit of vector G going upwards; it's positive 2.80 kilometers times sin of 10.0 degrees— sin because it's the opposite leg of this triangle that we are finding— and all this works out to negative 6.5701 kilometers. And these numbers make sense based on our drawing, you know, our drawing isn't perfect but it should at least give us an idea of whether something is positive or negative. We expect the x component of the resultant to be positive and the y component of the resultant to be negative and the y component should be of greater magnitude than the x component and that's what we see here— the y components are roughly double in magnitude to the x component. Okay! Always good to do a little reality check as you go along. The magnitude of the resultant then is the square root of the sum of the squares of the components. So that's square root of 3.2799 kilometers squared plus negative 6.5701 kilometers squared and that works out to 7.34 kilometers. The next thing to specify our vector is to also find its direction so we will find this angle θ. And this angle θ we know is here with respect to the x axis— this is the angle θ that we want to find for the resultant— and we can see its direction based on our picture and so we don't need to think about negative signs because the purpose of negative signs is to indicate direction but we can see direction from our picture so we are going to concern ourselves only with the magnitudes of the y and the x components of the resultant for determining the angle. So we take the inverse tangent of the opposite leg of this triangle— that is the magnitude of the y component divided by the magnitude of the x component. So it's the inverse tangent of 6.5701 kilometers divided by 3.2799 kilometers and that's 63.5 degrees and this is towards the south of east... we can see from our picture that it is to the south compared to east. Okay! So the final answer is that the resultant is 7.34 kilometers, 63.5 degrees S of E— south of east.