Question
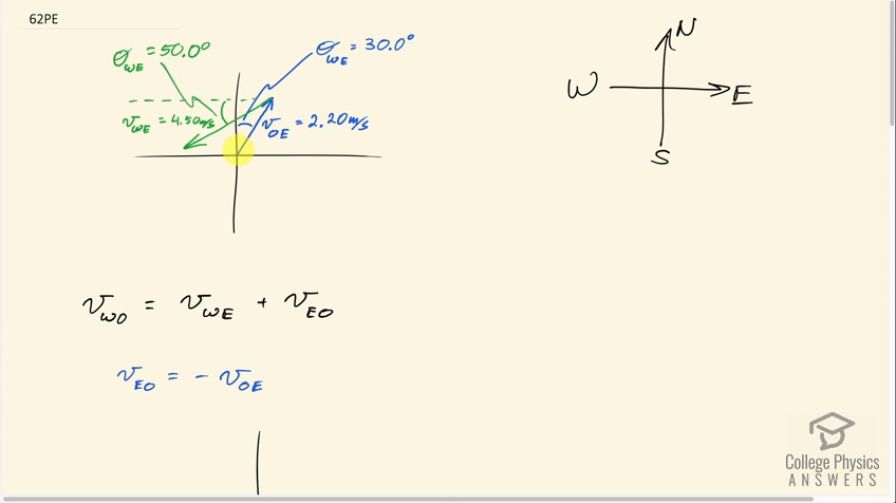
The velocity of the wind relative to the water is crucial to sailboats. Suppose a sailboat is in an ocean current that has a velocity of 2.20 m/s in a direction east of north relative to the Earth. It encounters a wind that has a velocity of 4.50 m/s in a direction of south of west relative to the Earth. What is the velocity of the wind relative to the water?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 3, Problem 62 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
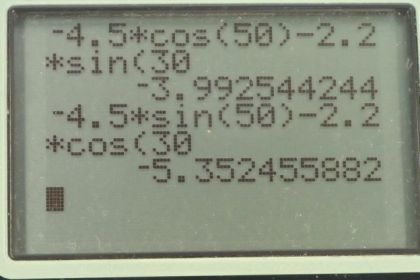
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're gonna figure out the velocity of the Wind with respect to the Ocean in this question. So what we're given is the velocity of the Ocean with respect to the Earth. This is the current and it's 2.2 meters per second, 30 degrees to the east compared to north. And then there's also the velocity of the Wind with respect to the Earth, which is 4.5 meters per second 50 degrees to the south of west. So this is a drawing of both those vectors, but we're actually not gonna take the resultant of these two as their drawn here. Instead, let’s consider the addition of relative velocities with regards to this pattern of subscripts. What we want is to know the velocity of the Wind with respect to the Ocean. So to find that we can add the velocity of the Wind with respect to the Earth to the velocity of the Earth with respect to the Ocean. And when we have it set up so that the inner subscripts are the same. They're both e for Earth in this case. That means they will cancel. And we’ll be left with the outer subscript velocities here. So we have w and o, and so this will find us the velocity of the Wind with respect to the Ocean. So we're given this term conveniently right here. But this term we're not given explicitly, we’re given the velocity of the Ocean with respect to the Earth. What we want is the velocity of the Earth with respect to the Ocean. Now to have these subscripts flipped around you just need to put a negative sign in front. So this velocity that we want is going to be the negative of the velocity of the Ocean with respect to the Earth that we're given. And to take the negative of a vector is to take the same vector but in the opposite direction. So what we really have is this picture here. This is the velocity of the Earth with respect to the Ocean in the opposite direction to the Ocean with respect to the Earth. So that's 30 degrees to the west compared to south. And we’ll add to that using the head to tail method, the velocity of the Wind with respect to the earth. And the e subscripts are both inner subscripts, they cancel. And we’re left with w and o. And that's what this would be this resultant, the velocity of the Wind with respect to the Ocean. So we’re gonna find this angle here to the south of west, and we’ll find this magnitude of this speed here. Okay, so the x-component of the velocity of the Wind with respect to the Ocean; will be the sum of the x-components of these two vectors. And so the Wind with respect to the Earth, it's x-component is to the left. And so that's why we have this minus sign here. And along this dotted line is the adjacent leg compared to this angle here. And so we have cosine of theta w e multiplied by this hypotenuse. And I'm referring to this triangle here when I say that. So this is the x-component and this is the y-component for the Wind with respect to the Earth velocity. Okay, and then with regards to the Earth with respect to the Ocean velocity. We are finding the opposite leg of this blue triangle. Here's the blue velocity triangle, and this opposite component here, we’ll find by using the sine of this angle multiplied by the hypotenuse. And that is to the left again, like this, and so that's why there's a minus sign here. So we have negative 4.5 meters per second times cos 50, minus 2.2 meters per second times sine 30. Giving negative 3.9925 meters per second, is the x-component of the velocity of the Wind with respect to the Ocean. And then for the y-component, it’s the same idea, we have to look at these triangles to find out how to find the y-components. So we're gonna use the sine function for the green triangle. And we’ll use the cosine function for the blue triangle and both of these y-components are directed downwards. So they're both negative. So negative and negative. So we have negative 4.5 times sine of 50 minus 2.2 times cos of 30. Giving negative 5.3525 meters per second. So we're gonna combine these two components using Pythagoras to get the magnitude of the resultant velocity; So that’s the square root of negative 3.9925 meters per second squared, plus negative 5.3525 meters per seconds squared. Giving 6.68 meters per second. And then the direction will be the inverse tangent of the y-component divided by the x-component. I'm not concerned with negative signs here, because I know that we're finding this angle here. So the direction is taken care of by looking at the picture and knowing that this is to the south compared to west. Then you see this cardinal directions here an angle that's here is to the south compared to west. So we taken the inverse tangent of the y-component divided by the x-component. And that gives 53.3 degrees, so our final answer for the velocity of the Wind with respect to the Ocean is 6.68 meters per second, 53.3 degrees south of west.