Question
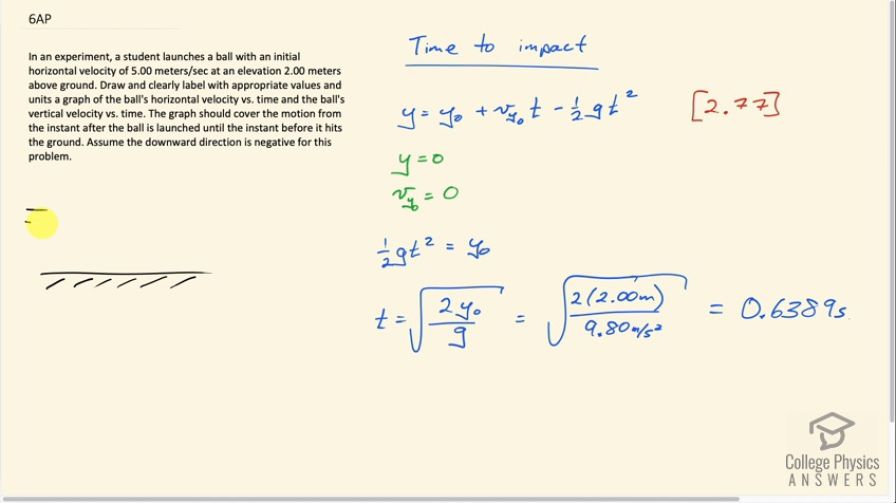
In an experiment, a student launches a ball with an initial horizontal velocity of 5.00 meters/sec at an elevation 2.00 meters above ground. Draw and clearly label with appropriate values and units a graph of the ball's horizontal velocity vs. time and the ball's vertical velocity vs. time. The graph should cover the motion from the instant after the ball is launched until the instant before it hits the ground. Assume the downward direction is negative for this problem.
Final Answer
Please see the solution video and the Google Sheets spreadsheet: https://drive.google.com/open?id=1MflnD2toyXjJE4e159y83QSRa55pYZWiBqZ75qtGVzo
Solution video
OpenStax College Physics for AP® Courses, Chapter 3, Problem 6 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A ball begins at an elevation of two meters above the ground and maybe it's being shot out of some kind of spring-loaded device maybe and shoots out with a speed horizontally of 5.00 meters per second. Then it'll be in free fall as well and so it's going to have a vertical acceleration due to gravity. It begins at a height of two meters, which we'll write down here. That means to say that the final position is zero and the initial position, y naught equals two meters. The initial y-component of its velocity is zero. We're going to draw graphs of its horizontal velocity with time and its vertical velocity with time. In order to figure out how much time we should have in our column for time, we'll figure out how long it takes for it to hit the ground starting at height of 2 meters. Equation 77 in Chapter 2 says this, the final y position equals the initial y position plus the initial y velocity times time minus one half times acceleration due to gravity times time squared. But given that the final position is zero and the initial y velocity is zero, we can have just these two terms. We'll move this one to the left-hand side by adding one-half gt squared to both sides. Then we get this line, and then we'll multiply both sides by 2 over g. Then we'll end up with t squared equals y naught times 2 over g and then we'll take the square root of both sides as we solve for time. Time is square root of 2 times 2 meters divided by 9.8 meters per second squared, which is 0.6389 seconds. That's what we need for our maximum time approximately in our spreadsheet. I'm using Google Docs here, and here's the horizontal velocity with time. Because there's no acceleration horizontally, we just have five arrows for every single time. We started at zero seconds, and 0.1, 0.2, 0.3 up to 0.7, and the horizontal velocity is always 5. This is just a straight line horizontally. That's all there is to that. Our graph has units on the axis, and seconds here for time, and meters per second for the velocity, and a descriptive title. Now the white position is a little bit more interesting. We can make an equation for the y velocity. It's going to be the initial y velocity, which we've already said is zero, minus acceleration due to gravity times time. Essentially becomes this once you substitute for zero for Vy naught and this is the formula that I'm putting in the spreadsheet for the y-direction. You can see that formula here. It's negative 9.8 times whatever the time is in the cell in the column A with the same row, which is for in this case. Here, we have negative 9.8 times the time in A5, in A6, in A7, and so on. They made a graph of that and we can see that this is a straight line showing that the velocity is increasing linearly with time, which we expect because this is a graph with a slope of negative g, where it's a slope-intercept form where y equals mx plus b where b is zero. And so this is a form y equals mx, where m being the slope is negative b. There we go.
