Question
Prove that the trajectory of a projectile is parabolic, having the form . To obtain this expression, solve the equation for and substitute it into the expressions for (These equations describe the and positions of a projectile that starts at the origin.) You should obtain an equation of the form where and are constants.
Final Answer
Please see the solution video
Solution video
OpenStax College Physics for AP® Courses, Chapter 3, Problem 48 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
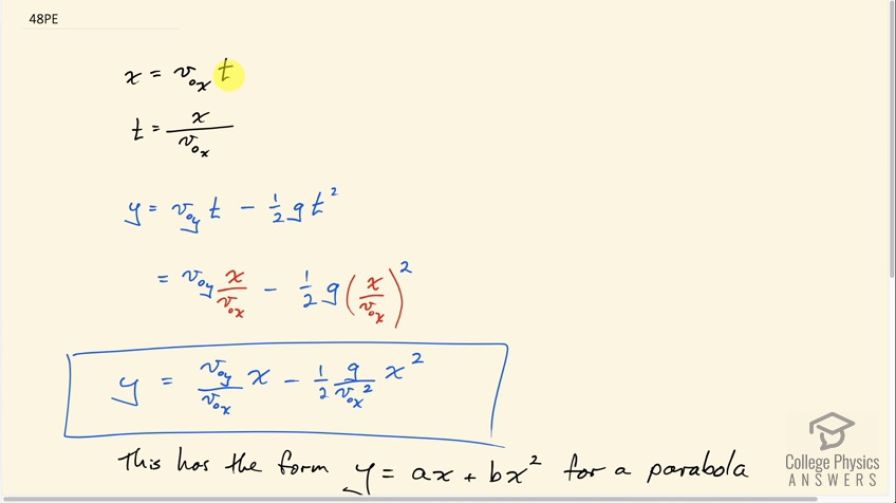
This is College Physics Answers with Shaun Dychko. The x-position of a projectile is the initial x-component of its velocity, multiplied by time. And we can solve this for t, by dividing both sides by v naught x. In which case t is x over v naught x. And that can be substituted in for t, in this expression for the y-position. So the initial y-component of its velocity times time, minus one half g t squared is the y-position. And will replace t with x over v naught x in both cases here. Then if we clean this up a little, we have v naught y x over v naught x, minus one half g over v naught x squared, times x squared. Now this is the form of a parabola because it has a form some constant A, multiplied by the variable to the power of one. Plus some other constant, multiplied by the variable to the power of two. And these are constants because these are the initial components to its initial velocity. So that's just happens that one moment that the, when it's first launched. And so this factor A, the coefficient of the linear term is v naught y over v naught x. And the coefficient of the quadratic term is negative g over two v naught x squared. And both of these are constants, and so we have shown that this is a parabola.