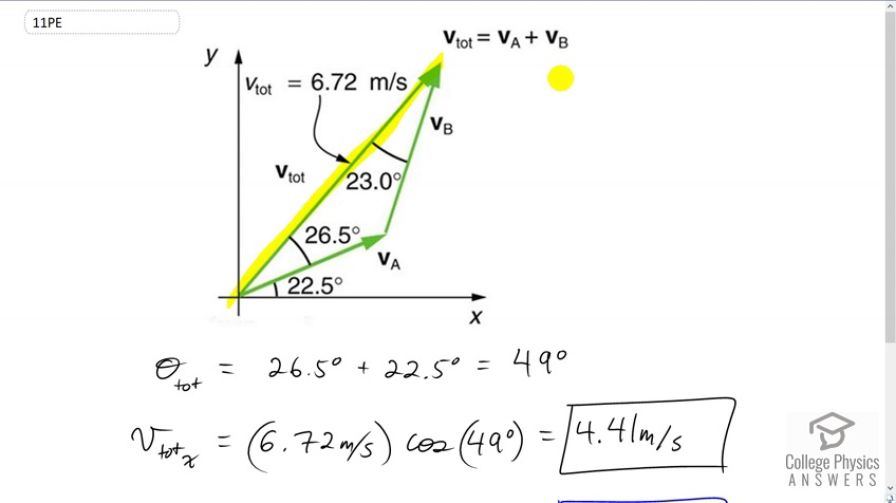
Question
Find the components of along the and axes in Figure 3.57.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 3, Problem 11 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Our job in this question is to find the x and y components of this vector, the total vector, the resultant of Va plus Vb. It has components along the x axis, here's the x component of the vector and then here is the y component of the vector. Right here, this is V total y component, the amount by which the resultant goes up. Then along here horizontally, we have V total x, the amount by which this yellow vector goes to the right. To find the x component, we're going to need to know what this angle is in here and then we'll use cosine multiplied by the hypotenuse to find the length of this adjacent leg of this right triangle. So to find this angle we have to add the 26 and a half to the 22.5. That gives us 49 degrees and so the x component of the total then is the length of the vector, 6.72 meters per second, multiplied by cosine of 49. That gives 4.41 meters per second. They component of the V total is going to be 6.72 meters per second multiplied by sine of 49 degrees, and we're using sine because this is the opposite leg of the triangle. We use sine always to get the opposite leg of a triangle, multiplied by the hypotenuse and that gives 5.07 meters per second.
