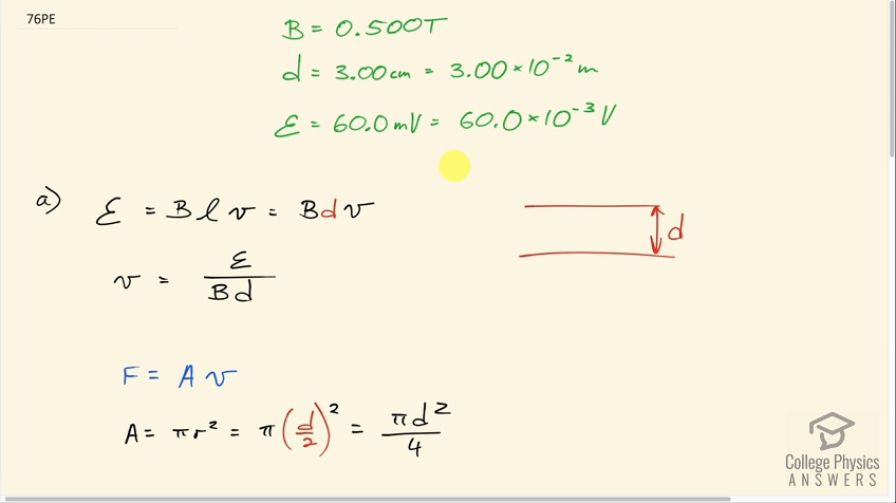
Question
To construct a nonmechanical water meter, a 0.500-T magnetic field is placed across the supply water pipe to a home and the Hall voltage is recorded. (a) Find the flow rate in liters per second through a 3.00-cm-diameter pipe if the Hall voltage is 60.0 mV. (b) What would the Hall voltage be for the same flow rate through a 10.0-cm-diameter pipe with the same field applied?
Final Answer
Solution video
OpenStax College Physics, Chapter 22, Problem 76 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A pipe with a diameter of 3.00 centimeters— this is the diameter of the pipe— has some water flowing through it and this water has some dissolved ions in it, as most water does, and those charged ions can form a charged distribution when a magnetic field is put across this pipe. So if a magnetic field is directed into the page here say then putting your fingers in the direction of that magnetic field and your thumb in the direction of motion of the water—let's say the water's to the right— then you would have the positive ions pushed to the top and the negative ions pushed to the bottom of the pipe and so this causes a voltage and this is the Hall voltage that will be measured and it's 60 millivolts and the magnetic field strength here is 0.500 tesla and we are going to figure out what is the speed of the water in the pipe? This is a non-mechanical water flow rate measuring device. So the Hall voltage is magnetic field times the diameter of the pipe times the speed of the water in it; usually the formula has letter l in here but we can substitute the letter d... it's nothing significant about that, I am just putting in this different letter because it's diameter in this case. We have to solve for v: we'll divide both sides by Bd so the speed of the water then is the Hall voltage divided by the magnetic field strength times the diameter of the pipe. Now the volume flow rate through a pipe is its cross-sectional area multiplied by the speed of a fluid and since this is a pipe with a circular cross-section, its area is going to be π times its radius squared. But we don't know the radius but we do know the diameter and so the radius is half the diameter so we substitute d over 2 in place of r and we end up with πd squared over 4 is the area. So we can substitute that in for A here and then we substitute this in for v and now we have a formula for the volume flow rate. So this d squared divided by d is d to the power of 1 so we have π times the Hall voltage times the diameter of the pipe divided by 4 times the magnetic field strength. So that's π times 60 millivolts written as times 10 to the minus 3 volts times 3.00 times 10 to the minus 2 meters— diameter of the pipe— divided by 4 times 0.500 tesla and its 2.8274 times 10 to the minus 3 cubic meters per second. And the question is asking for the volume flow rate in liters per second so we will have to do a conversion but first let's just verify that this does work out to cubic meters per second because that isn't obvious from units in the formula, we have volts times meters divided by tesla. So we have volts times meters divided by tesla writing. you know, dimensional analysis in square brackets like this and the volts you can get from this E equals Blv we can get different units for volts by examining this formula and so voltage can be thought of as tesla times meters times meters... per second I should say so tesla times meters times meters per second, this gives cubic meters per second and then tesla divided by tesla, which cancels so we have cubic meters per second or the dimensions of this here. Then we take this answer, multiply it by 1000 liters for every cubic meter and we get 2.83 liters per second is the volume flow rate. Then part (b) asks what would the Hall voltage be for the same flow rate through a 10.0 centimeter diameter pipe? So we expect the Hall voltage to reduce because increasing the diameter will reduce the speed given the same volume flow rate. So if we put the same number of liters through the pipe per second as before now that we have a larger diameter to push that water through, we need it to go more slowly to have the same volume going through per time. So we repurpose this formula from before where we say that the volume flow rate is π times the Hall voltage times the diameter divided by 4 times the magnetic field strength; I have put a subscript on this and this to indicate that they are different now for part (b)— a new Hall voltage that we have to find— and a new diameter that we are given 10.0 centimeters everything else is the same so the same volume flow rate, the same magnetic field so they do not get subscripts on letters F nor B. We are solving for the Hall voltage so multiply both sides by 4B over π times d 2 and we have then that the Hall voltage in the second case is 4 times the magnetic field strength times the volume flow rate divided by π times the new diameter of the pipe. So that's 4 times 0.500 tesla times 2.8274 times 10 to the minus 3 cubic meters per second divided by π times 10.0 times 10 to the minus 2 meters. Notice we use the version of this volume flow rate in units of cubic meters per second because this is an mks unit— meters, kilograms and seconds— rather than the liters per second. This works out to 18.0 millivolts, which indeed is less than the 60 millivolts that we had with the small diameter tube.
