Question
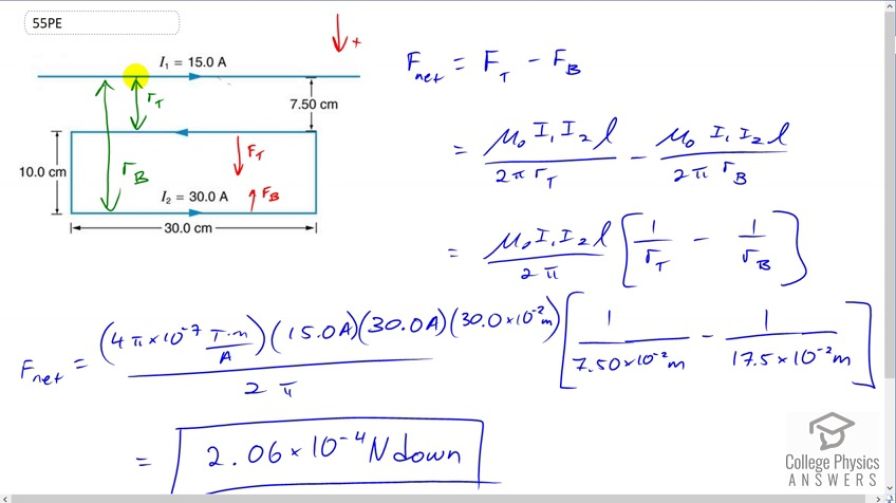
Figure 22.57 shows a long straight wire near a rectangular current loop. What is the direction and magnitude of the total force on the loop?
Final Answer
Solution video
OpenStax College Physics, Chapter 22, Problem 55 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a current carrying wire here, and a loop carrying a current here. The question is what force will this current carrying wire on the top exert on the bottom loop. Now there’s going to be forces on all four sides of this rectangle and let’s figure out what direction those forces will be. So consider what magnetic field is being created by this top wire first of all, we’ll grab this wire with our right hand, with our thumb pointing to the right in the direction of the current, and then the direction our fingers are pointing when we place them in this location is downwards into the page. So the magnetic field created by this top wire at the position of this loop is down. So each of these segments or these sides of this rectangle are in the presence of a magnetic field pointing down into the page. So the next question is what force will this magnetic field exert on the current that’s flowing in this loop. So let’s consider this top side here, and I’ve got the answer here already but let’s figure out how I got this answer. We will, first of all put our thumb in the direction of the current which is to the left, the fingers go into the page in the direction of the magnetic field, and our palm is pointing in the direction of the force, and that would be downwards, our palm is facing down. So I’ve labelled this F subscript T for top, the force on the top side. And we’re gonna take downwards to be the positive direction in this case and we’re gonna find a net force by taking the force on the top minus the force on the bottom because the force on the bottom is going to be going upwards. And we’ll see why by pointing our thumb to the right because that’s the direction of the current in this bottom side, and our fingers point into the page and our palm is facing upwards. And I’ve drawn this arrow a little bit shorter than the force on the top because the magnetic field strength here will be smaller since this is a further distance from the wire. And so we can see already from our vector diagram that we’re going to have a net force downwards because the force on the top will be greater down than the force on the bottom is upwards. Now on the left and right sides, I didn’t draw any arrows there because these forces are going to be towards each other and cancel each other out. So let’s verify that to be sure. So in this segment of wire, we have a current pointing down so our thumb goes downwards, the fingers go into the page in the direction of magnetic field, and the palm is to the right, and so there is a force to the right here. I guess I’m drawing forces in red here aren’t I. So that’s the force on the left side. And then over here, we have a current going upwards and so our thumb points up, our fingers point into the page, and our palm faces to the left. And so this is a force on the right hand segment. And these forces are going to be of equal magnitude, because they have the same gradient of magnetic field over their length and they contain the same current, so we expect no net force at all on the left and right sides of this rectangle. Alright. So the net force will be entirely in the y direction, so it’s going to be the top force minus the bottom force, taking down to be positive. And so we have permeability of free space times the product of the two currents times the length of the wire segment divided by two pi times the distance to the top wire, r subscript T for top, and then subtract from that the force on the bottom one which is mu naught I1 I2 l over two pi r subscript B because it’s the bottom segment of wire. And the l does not need a subscript because the top and bottom are of equal length in this rectangle. And we can factor out all these common factors and we’re left with mu naught I1 I2 l over two pi times one over rT minus one over rB. So then we plug in numbers and we get four pi times ten to the minus seven Tesla meters per Amp times 15 Amps in the top wire times 30 Amps in the bottom wire, times 30 times ten to the minus two meters which is 30 centimeters, divided by two pi, times one over 7.5 times ten to the minus two meters, the distance to the top side of the rectangle, minus one over the distance to the bottom side of the rectangle which is 7.5 plus ten, so that’s 17.5 times ten to the minus two meters. And this works out to 2.06 times ten to the minus four newtons, and this is downwards.
