OpenStax College Physics, Chapter 22, Problem 19 (Problems & Exercises)

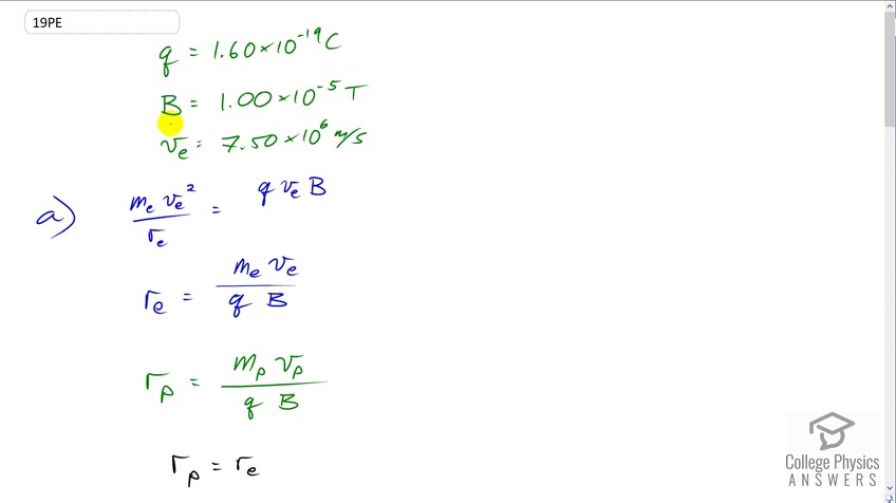
This is College Physics Answers with Shaun Dychko. In question 19 we had an electron moving with a speed of 7.5 times ten to the six meters per second perpendicular to a magnetic field of strength one times ten to the minus five Tesla. The charge on the electron is the ometry charge of 1.6 times ten to the minus 19 Coulombs. In this question, the charge would be of the same magnitude because we're dealing with a proton and the speed however will be different in different parts of the question. So I did put a subscript on the speed here. This is speed of the electron from question 12. And the magnetic fields are the same for both problems so it does not need a subscript. Now we're told in part a that the radius of the circular path in which the electron went in question 12 is the same as the radius on the circular path for the proton in this part of the question part a. So let's create expressions for the radius of the path and we know that centripetal force is going to be provided by the magnetic field. And this is the force due to the magnetic field, charge times speed times magnetic field strength. And so q times the speed of the electron times magnetic field equals the mass electron times speed electron squared divided by its electron's radius, the radius of its path that is. And we'll solve this for re by multiplying both sides by re over qveB and we get that the radius of the path of the electron was the mass electron times its speed to the power of one divided by qb. Now we could write down the same equation using subscripts P for the proton in this question. And we would end up with the same kind of result but just with a P instead of an E, so I've written that here. And let's equate to these two fractions because we're told the radii of the circular paths are meant to be the same. And so that means that mpvp over qB equals meve over qB. And then we'll isolate vp. We'll solve for the speed of the proton given that the radius of its circular path is the same as that of the electron from question 12. So we'll multiply both sides by qB over mass of the proton and we end up with vp is the ratio of the mass of the electron to the proton multiplied by the speed of the electron. So that's 9.11 times ten to the minus 31 kilograms times seven and a half times ten to the six meters per second speed of electron divided by mass of the proton which is 1.67 times ten to the minus 27 kilograms which is 4090 meters per second. And so we can see that the speed of the proton is much lower than the electron was and that's because the mass of the proton is so much greater than the mass of the electron. In part b, the radius that the proton would have if it was going the same speed as the electron was in question 12 would be this formula here. So we have the mass of the proton multiplied by its speed which is that of the electron from question 12 divided the charge in the proton times the magnetic field strength. And this gives 7830 meters. And in question c, we're told that the kinetic energy of the proton is the same as the kinetic energy the electron had in question 12. We'll solve this for vp and we'll multiply both sides by two over mp. And then take the square root of both sides and square root of ve squared is just ve. So we have square root of me over mp times ve and substitute that in for the speed of the proton here. We're using this formula a lot here. So replacing vp with this here and mp divided by square root mp is square root mp. And so we simplify it to this equation here. And so the square root of the product of mass is times speed of the electron divided by qBis going to be the radius of the proton's path. So that's square root times mass of the proton times mass of the electron times the speed of the electron had in question 12 divided by the charge in the proton times magnetic field strength. And this gives 183 meters. Then in question d we're told that the momenta are the same. The momentum of the proton in this question is the same that the momentum of the electron had in question 12. And we'll solve for what vp is now. And so it's the ratio of the masses times ve. And so we'll substitute that in for the speed of the proton. And the mp cancels and we're left with the radius of the proton's path is mass electron times speed of the electron divided by qB. And so that's 9.11 times ten to the minus 31 kilograms mass of the electrons times the speed of the electron for question 12 divided by 1.6 times ten to the minus 19 Coulombs times one times ten to the minus five Tesla which is 4.27 meters. And that's the same radius that the electron would've had in question 12.