OpenStax College Physics, Chapter 22, Problem 16 (Problems & Exercises)

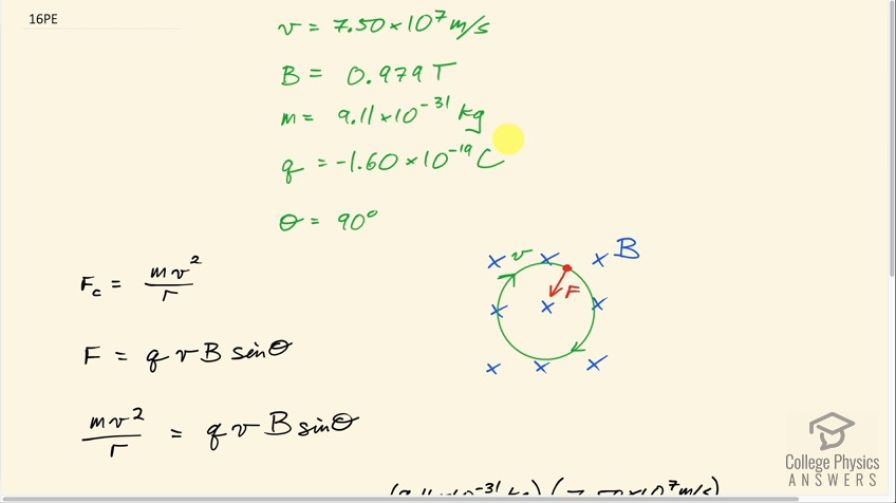
This is College Physics Answers with Shaun Dychko. This question asks what radius— circular path— does an electron travel if it moves at the same speed and the same magnetic field as the proton in exercise 13? So we need to look up what the magnetic field was in that question and so the answer to that question was 0.979 tesla; the speed of the proton in that question was 7.50 times 10 to the 7 meters per second. Now because we are dealing with an electron here, the mass is gonna be 9.11 times 10 to the minus 31 kilograms and the elementary charge is 1.60 times 10 to the minus 19 coulombs. So here's the picture that we are dealing with and the question is what is the radius of this circle that the electron is traveling in? Let's verify that the picture is right... we will practice our right-hand rule first by putting fingers of our right hand into the page in the direction of these magnetic field lines and then our thumb will go in the direction of the velocity and our palm is facing away from the center which will be the direction of a positive charge but because this is a negatively charged electron, the force direction is the opposite to our palm and that is towards the center of this circle. So this Lorentz Force is the charge multiplied by its speed times the magnetic field strength times sin of the angle between the velocity and the magnetic field and this is going to be supplying the centripetal force which has an expression for any particle moving in a circle, which is its mass times its speed squared divided by the radius and this velocity and this magnetic field are perpendicular because if you were to tilt this picture such that you bring this end towards you and this end away from you then you would have this side view and you would have magnetic field straight down and velocity to the right —if you were to look at this end of the circle anyhow— whether you say it's to the right or the left kinda depends on which end of the circle you are on but needless to say, either way the velocity is moving in this sort of line— one direction or the other— it's moving to the right at this point and it's moving to the left at this point. But the main point is that they are perpendicular— magnetic field and velocity. Good! So then the centripetal force then equals this Lorentz Force and you can solve for r by multiplying both sides by r divided by qvBsin Θ and then switch the sides around and v squared divided by v is v to the power of 1 so we have the radius then is mass times speed divided by charge times magnetic field strength times sin Θ. So that's 9.11 times 10 to the minus 31 kilograms—mass of an electron— times its speed of 7.50 times 10 to the 7 meters per second divided by the elementary charge times 0.979 tesla times sin of 90 degrees and that's 4.36 times 10 to the minus 4 meters is the radius of its path.