Question
A proton has a magnetic field due to its spin on its axis. The field is similar to that created by a circular current loop 0.650×10−15 m0.650 \times 10^{-15} \textrm{ m} in radius with a current of 1.05×104 A1.05 \times 10^4 \textrm{ A} (no kidding). Find the maximum torque on a proton in a 2.50 T2.50 \textrm{ T} field. (This is a significant torque on a small particle.)
Question by OpenStax is licensed under CC BY 4.0
Final Answer

3.48×10−26 N⋅m3.48 \times 10^{-26} \textrm{ N}\cdot\textrm{m}

Solution video

OpenStax College Physics, Chapter 22, Problem 47 (Problems & Exercises)

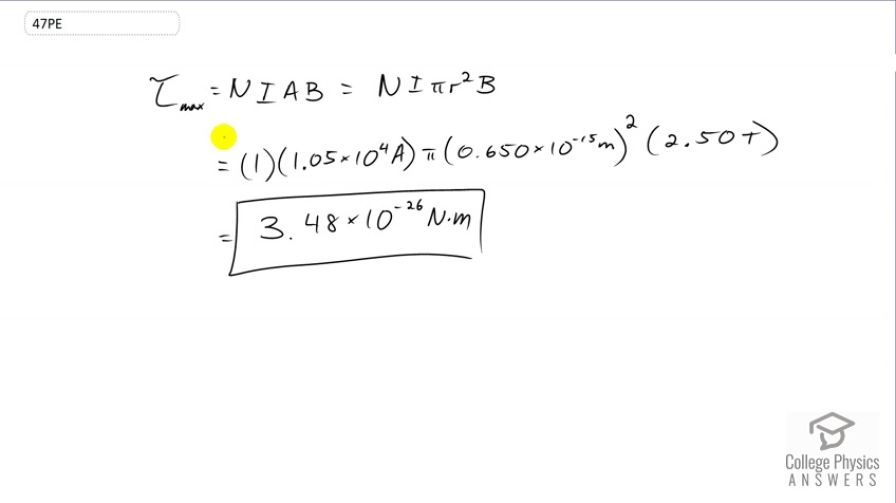
OpenStax College Physics, Chapter 22, Problem 47 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 22, Problem 47 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. We're told that a single proton has a magnetic field due to its spinning on its access equivalent to that created by a tiny tiny circular current loop with a given current. And the current loop who have an area equal to Pi times its radius squared. And so we substitute that in for A in this formula for the torque. This is the maximum torque. The sine theta term is gone because you get the maximum torque when you have theta equal to 90. In which case, sine theta is one. And so we have one loop because it's just one proton. And we have other things given to us like the size of the loop and the current such that only one loop is needed, and times by the current of 1.05 times ten to the four Amps times by Pi times 0.6 times ten to the minus 15 meters which is 0.65 Femto meters and we square that and times by two and a half Tesla. And this works out to 3.48 times ten to the minus 26 Newton meters of torque.