Question
Find the range of visible wavelengths of light in crown glass.
Final Answer
Solution video
OpenStax College Physics, Chapter 27, Problem 2 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
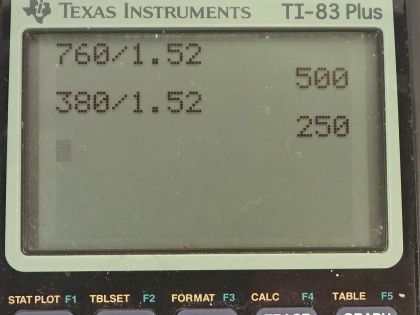
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The wavelengths in a vacuum of visible light range from 380 nanometers up to 760 nanometers we know what the index of refraction is for crown glass and the wavelength in the medium is the wavelength in the vacuum divided by the index of refraction. So the wavelengths that are possible in the crown glass will range from the minimum wavelength in a vacuum— 380 nanometers— divided by the index of refraction up to the maximum wavelength in a vacuum that's visible divided by the index of refraction. So that's 380 divided by 1.52, which is 250 nanometers up to 760 nanometers divided by 1.52, which is 500 nanometers. So wavelengths that are visible in this range will exist in the crown glass.