Question
The headlights of a car are 1.3 m apart. What is the maximum distance at which the eye can resolve these two headlights? Take the pupil diameter to be 0.40 cm.
Final Answer
Solution video
OpenStax College Physics, Chapter 27, Problem 66 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
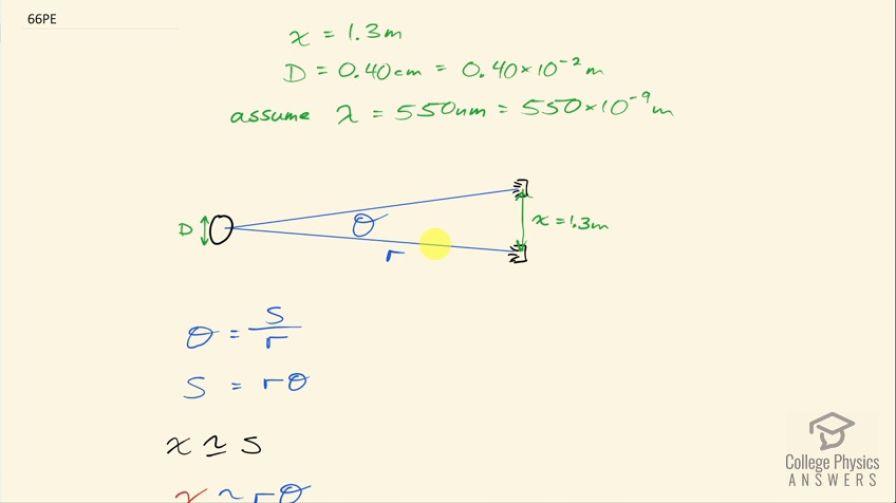
This is College Physics Answers with Shaun Dychko. The headlights of a car are 1.3 meters apart and the diameter of the eye of a person looking at these headlights is 0.40 centimeters and we want to know what is the maximum distance away that these headlights can be and this person can still distinguish them as two distinct headlights? We'll need to know what this angle is here— that's from Rayleigh's Criterion— and we also need to make an assumption about what the wavelength is of the light and we'll assume that it's in the middle of the visible spectrum at 550 nanometers. So I converted these things into meters and let's talk about how this distance x is related to the arc length of a circle that you could imagine being created with this as the radius— it's not a very good circle but you can imagine there's some arc length here— and Θ measured in radians is the arc length, which we'll call s suppose... s goes from one headlight to the other along this curve of this circle it's s divided by the radius of the circle r in which case we can solve for s by multiplying both sides by r and get that the arc length is the radius times this angle in radians but now since this angle is going to be so small if you were to zoom in on this portion of a circle really this arc length is going to be about the same as the straight line distance from one headlight to the other. So we are making an assumption then in the case of a small angle here that this distance x is the same as the arc length s in which case we'll say that x equals approximately the radius times the angle. So we can divide both sides by Θ to solve for r which is this distance from the eye to the headlights and r is going to be approximately x over Θ. So Θ we can find from Rayleigh's Criterion: it's 1.22 times the wavelength divided by the diameter of the pupil. So that's 1.22 times 550 times 10 to the minus 9 meters divided by 0.40 times 10 to the minus 2 meters which is 1.6775 times 10 to the minus 4 radians. So the distance then to the car is 1.3 meters divided by that angle and that is 7.75 kilometers.
