OpenStax College Physics, Chapter 27, Problem 62 (Problems & Exercises)

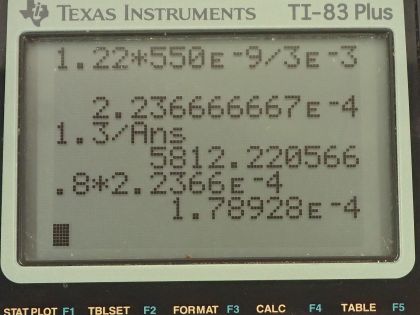
This is College Physics Answers with Shaun Dychko. We are going to find the angle between two just barely resolvable distinct points considering the pupil of the eye is 3.00 millimeters in diameter and the average wavelength of visible light is 550 nanometers. So the angle, The Rayleigh Criterion in other words, is 1.22 times the wavelength divided by the pupil diameter. So that's 1.22 times 550 times 10 to the minus 9 meters divided by 3.00 times 10 to the minus 3 meters and that is 2.24 times 10 to the minus 4 radians. Part (b) asks given this limit to the eye, what is the greatest possible distance away that a car could be where you can still distinguish between the two headlights assuming the headlights are separated by 1.30 meters? So we have this angle that is the smallest possible angle between the headlights for given this certain pupil diameter here and we are going to figure out what r is the radius of an imaginary circle that's like this where this is the center and this is the radius and this distance x is approximately the same as the arc length of this portion of the circle and that arc length is defined to be... well, sorry the angle Θ in radians is defined to be this arc length s— between these two headlights here— divided by the radius of the circle and with such a small angle, the arc length is approximately the same as this straight line distance between these two points the cord is the word for it in the technical, geometrical term for a line connecting to parts of a circle without going through the center. Okay! So this distance x is approximately the same as the radius times this angle because you can solve for the arc length here by multiplying both sides by r and say that the arc length is r times Θ and this arc length is approximately the same as the straight line distance and so we'll say x is approximately equal to radius times the angle. Okay! So we'll solve for r then by dividing both sides by the angle. So r is this straight line distance divided by the angle that's 1.30 meters divided by 2.2367 times 10 to the minus 4 radians and that is 5.81 kilometers. So if the car is further away than 5.81 kilometers, you would not be able to distinguish between the two different headlights if it just looked like one single light. For part (c), we want to find the distance between two barely resolvable points held at arms length. So we know what this angle is that's the angle in part (a) this minimum angle given the average wavelength of light and the size of the pupil and we are told that this distance between the eye and your hand is arms length, which is 0.800 meters and we have to figure out what x is so x is approximately equal to this radius of this imaginary circle multiplied by the angle. So that's 0.800 meters times 2.2367 times 10 to the minus 4 radians which is 0.179 millimeters. And part (d) asks compare the answer to part (c) to your everyday experience. Now if we were to hold a ruler at arms length, we can notice whether or not it's possible to see the millimeter divisions on it and it is easy to distinguish between each of the tick marks and so it's probably possible to distinguish between tick marks that were only 0.2 millimeters apart.