Question
(a) As a soap bubble thins it becomes dark, because the path length difference becomes small compared with the wavelength of light and there is a phase shift at the top surface. If it becomes dark when the path length difference is less than one-fourth the wavelength, what is the thickest the bubble can be and appear dark at all visible wavelengths? Assume the same index of refraction as water. (b) Discuss the fragility of the film considering the thickness found.
Question by OpenStax is licensed under CC BY 4.0
Final Answer
  1. 35.6 nm35.6 \textrm{ nm}
  2. The soap bubble will pop with the slightest breeze.

Solution video

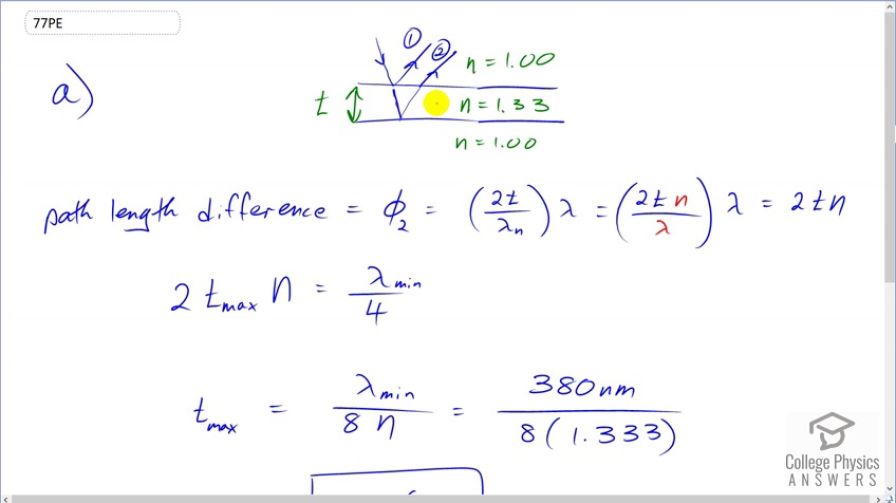
OpenStax College Physics, Chapter 27, Problem 77 (Problems & Exercises)

OpenStax College Physics, Chapter 27, Problem 77 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 27, Problem 77 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. This soap bubble that has an index of refraction of 1.33 the same as water is very thin and it's so thin that it will appear black because there will be destructive interference for all the different wavelengths when it's so thin. Because this path length difference won't be enough to cause constructive interference for any of the colors. And so there will be only destructive interference because this reflection at this interface between the air and the soap bubble was going to be phase shifted by lambda over two because it's going from a medium of low index of refraction 1.0 to a medium of high index of refraction 1.33 . So this pie over two phase shift occurs here and the path length difference due to Ray two travelling through the slope filming then reflecting off the bottom side of it that path length difference is not enough to cause any constructive interference. And so there will be only destructive interference this path length difference is insignificant. And so the phase difference between these two rays is essentially pie over two the phase shit due to Ray one. Now this path length difference is insignificant if it is less than a quarter of the wavelength we're told in the question. And so we need to make an expression for what this path length difference is and then we'll set it equal to a quarter of the wavelength. So path length difference which is a phase shift for Ray two is going to be 2 times the thickness and I have two times there because the ray goes once the thickness and then a second time back up and this fraction is the number of wavelengths. That's the number of lambdas because I'm dividing that total distance by the number of nanometres per wavelength. And so that gives the number of wavelengths and this is the wavelength within the soap bubble material. So that's why there's a subscript n there. To say that this is the wavelength in this medium of index refraction n and we have an expression for that wavelength n is the wavelength in a vacuum or air because air’s index of refraction is close enough to that of a vacuum divided by the index refraction n . And so having lambda divided by n is what this is. And dividing by it means multiplied by its reciprocal. So that's why multiplying by n over lambda here and at this point the lambda cancel. And the reason we're multiplying by lambda here s because this fraction gives us the number of lambdas and we're multiplying by the number of nanometres per lambda. And so for one lambda and then these lambdas cancel n little nanometres and so that gives us a path length difference and that expression for that then in the end is two times the thickness times the index of refraction of the soap film. Now all of this is meant to equal the wavelength divided by four. Now the question says it shall be dark for all visible wavelengths and so we get to choose which wavelength to use there. And since we want to know what is the maximum possible thickness that it could have. We're going to choose the minimum visible wavelength. And so this is going to be the wavelength of Violet that we put there. If we had chosen red there then it's still possible for Violet to have constructive interference. So anyway so we're finding the maximum possible thickness of this soap film can have such that every single visible wavelength will appear black. So now we rearrange this by dividing both sides by 2n and we get lambda minimum over eight times index refraction is the maximum thickness that this bubble can have and still have the appearance of Black. So it’s 380 nanometres divided by eight times 1.333 which is 35.6 nanometres. Now that is a very thin bubble. And so it's going to pop with the slightest breeze.

Comments

Submitted by ts on Thu, 04/29/2021 - 21:13

link

Hello,

In this problem, wouldn't the path length difference just be 2t? I was under the impression that the difference in path length was just equal to twice the thickness of the thin film, and didn't depend on the index of refraction, n.

Thanks!

For your reference, I have copy/pasted a definition for path length difference as it relates to thin film interference that I found online: "For waves incident normal to the film, the path length difference is twice the film thickness (∆ℓ = 2t)" Is this the correct definition for path length difference? Based on how it sounds here, I wouldn't think to take the index of refraction into account for this value.

Hi ts,
This is an important question. What you're describing is the path length difference if you measured with a ruler. However, it isn't strictly the path difference that we're interested in. What we're interested in is the phase difference between the two waves. The difference in path length traveled causes a phase difference, sure, but it's the phase difference that we need to calculate. What's interesting is to know how much the wave that traveled through the thin film progressed during its journey down, then back up, through the thin film. The amount it progresses depends on its wavelength in the thin film. Consider a thought experiment: imagine that upon entering the thin film, the wave was stretched so that its wavelength became the same as the thickness. In this scenario, when that wave emerges from the top of the thin film to combine (interfere) with the wave that reflected directly from the top of the film, there would be constructive interference since the wave traveling through the thin film progressed exactly two wavelengths (once down, then again back up), so it would be in phase with the top-reflected wave. Crests would overlap and a drawing of the two waves would look exactly the same. In this thought experiment, I'm trying to illustrate that it doesn't matter what the thin film thickness is. The number of wavelengths progressed is what matters. The number of wavelengths progressed will be the wavelength in the film divided by the film thickness, and the wavelength in the film is λn\dfrac{\lambda}{n}.
Hope this helps,
Shaun

Hi
So based on your clarification above, shouldn't Φ be path length difference as stated on the video ? rather should Φ be the path length difference ?

Thank you
Eray

For the correction rather "should Φ be the phase difference ?"

Hi Eraycey, thank you for the question. I think the best terminology to use is whatever leads to a good understanding, and good problem solving. You're quite right that phase difference is what's important, but the phase shift of the ray that penetrates the thin film is due entirely to a path length difference compared with the ray reflected from the top of the film. The penetrating ray doesn't experience any phase shift due to reflection from the bottom interface between the thin film and air since at that interface its initial medium is of high index of refraction and the second medium is of low index of refraction. For the penetrating ray, phase shift and path length difference can be used interchangeably since in this particular circumstance the phase shift is due exclusively to the path length difference, so I don't think it's helpful (even though it isn't wrong) to say "phase shift" instead of "path length difference".
Hope this helps,
Shaun