OpenStax College Physics for AP® Courses, Chapter 25, Problem 21 (Problems & Exercises)

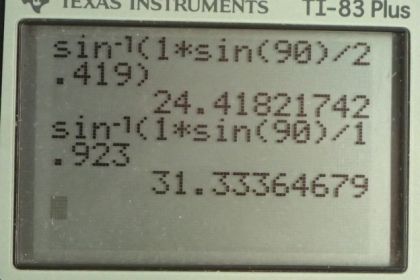
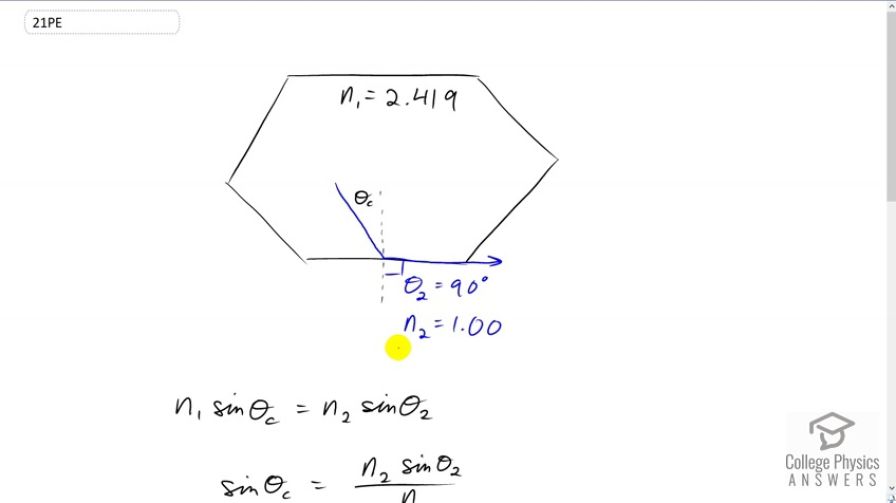
This is College Physics Answers with Shaun Dychko. We're going to verify that the critical angle for light going from diamond into air is 24.4 degrees. The critical angle is the angle — with respect to the normal, of course — that light will be refracted at 90 degrees just parallel to the interface between the two media. The media being diamond on the one hand, initially, and then air on the other hand. So, we're going to use Snell's law and say that the initial index of refraction, which is 2.419 for diamond, and say that the initial index of refraction, which is 2.419 for diamond, multiplied by sine of the critical angle of the angle of incidence, equals the index of refraction of the second medium, which is air, and so it has an index of refraction of 1.00, times sine of the angle of refraction, which is 90 degrees when we're at the critical angle. And then, we'll solve for theta here... theta c. So let's divide both sides by n one, to begin with. And then we get sin theta c equals n two sin theta two over n one. And then, take the inverse sine of both sides. And we get the critical angle the inverse sine of n two sine theta two over n one. And so, the second medium is air so it has an index of refraction of one, and we multiply by sine 90, which is one. And divide by 2.419 and take the inverse sine of all that and you get 24.4 degrees. Now, so here we have a formula for calculating critical angle, and we'll use that for...zirconium? Is that what it is? Or zircon. Zircon... Whatever that is. So we have an index of refraction for it anyway, given to us in that table 25.1. And plugging in 1.923 for the index of refraction of the initial medium, we get 31.3 degrees is the critical angle.