Question
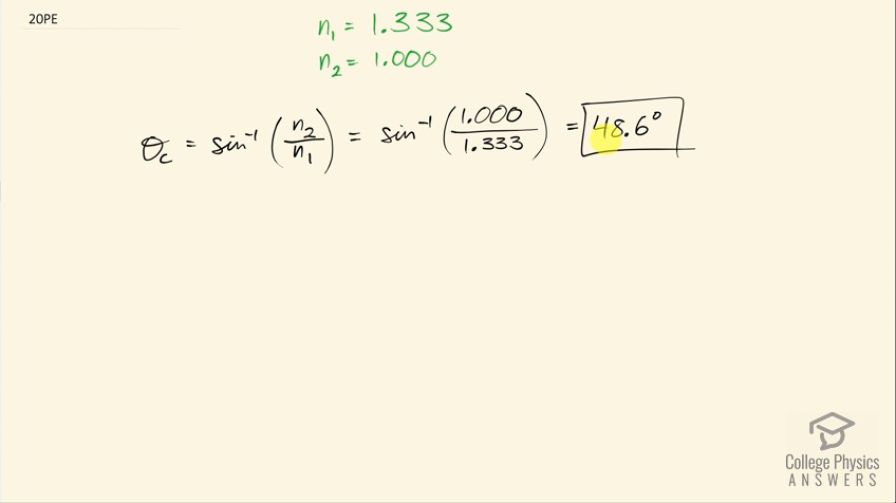
Verify that the critical angle for light going from water to air is , as discussed at the end of Example 25.4,
regarding the critical angle for light traveling in a polystyrene (a type of plastic) pipe surrounded by air.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 25, Problem 20 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to verify that the critical angle for a ray of light going from water into air is 48.6 degrees. So we label the water as n 1— the first index of refraction because that's where the ray begins— and the second medium is air and so we'll label that n 2 because it's the second medium and this angle here with respect to the perpendicular, it has to be 90 degrees when you have the critical angle for the incident angle. So the critical angle is the inverse sin of the second index of refraction divided by the first. So that's the inverse sin of 1, which is the index of refraction of air divided by 1.333—the index of refraction of water— and this is 48.6 degrees.
