Question
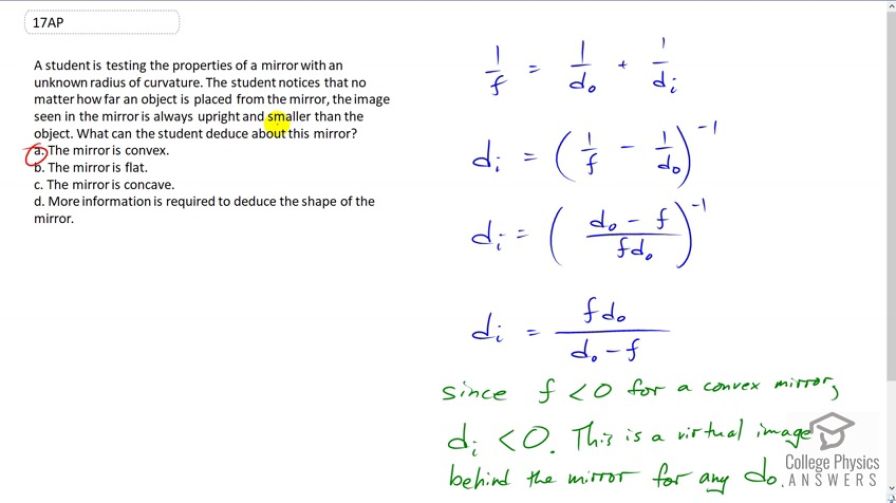
A student is testing the properties of a mirror with an unknown radius of curvature. The student notices that no matter how far an object is placed from the mirror, the image seen in the mirror is always upright and smaller than the object. What can the student deduce about this mirror?
- The mirror is convex.
- The mirror is flat.
- The mirror is concave.
- More information is required to deduce the shape of the mirror.
Final Answer
(a)
Solution video
OpenStax College Physics for AP® Courses, Chapter 25, Problem 17 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. A student notices no matter how far an object is placed from a particular mirror, the image is always upright and smaller. So, what can we figure out about this mirror? We're going to figure out that it's convex and we'll use this thin lens equation, which also applies to mirrors, to solve for the image distance and then talk about what could the focal lengths be. And then, based on knowing the focal length, the already conditions on the focal length, then we'll figure out what type of mirror it is. So, the one over the focal length is one over the object distance plus one over image distance, which we can then solve for image distance by subtracting one over object distance from both sides and then take the both sides to the exponent negative one. So, then the distance is one over focal length minus one over object distance all to the negative one. And then, we can write this as a single fraction by multiplying this by Do over Do and then multiplying this by F over F, and we end up with object distance minus focal length divided by the common denominator focal length times object distance. We flip that fraction and then find that image distance is F Do over Do minus F. So, there are two possibilities here. Either F is greater than zero or it's less than zero. If F was greater than zero, which corresponds to a concave mirror or a converging mirror, then it will be possible to find a value of the object distance such that the denominator would be zero, and in which case the image would be at infinity. And, that's not happening here because we're told that no matter what the object distance is, we're always getting an upgraded and smaller image. And so then, this must be a convex mirror then because in that case, the focal length is negative and this denominator will always be a positive number and the numerator will always be a negative number because we're going to be taking a positive object distance minus a negative focal length, so that's always going to end up being positive in the bottom and the top will always be negative, because we're multiplying a positive object distance by a negative focal length. And then, we're going to have a negative image distance all the time and that corresponds to a virtual image behind the mirror. Since we said that focal length must be less than zero, that means it has to be a convex mirror.