Question
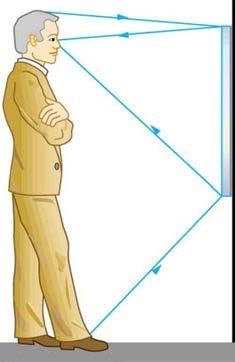
Suppose a man stands in front of a mirror as shown in Figure 25.49. His eyes are 1.65 m above the floor, and the top of his head is 0.13 m higher. Find the height above the floor of the top and bottom of the smallest mirror in which he can see both the top of his head and his feet. How is this distance related to the man’s height?
<b>Figure 25.49</b> A full-length mirror is one in which you can see all of yourself. It need not be as big as you, and its size is independent of your distance from it.
Figure 25.49 A full-length mirror is one in which you can see all of yourself. It need not be as big as you, and its size is independent of your distance from it.
Question by OpenStax is licensed under CC BY 4.0
Final Answer
hb=0.825 mh_\textrm{b} = 0.825 \textrm{ m} ht=1.72 mh_\textrm{t} = 1.72 \textrm{ m}

Solution video

OpenStax College Physics for AP® Courses, Chapter 25, Problem 1 (Problems & Exercises)

vote with a rating of votes with an average rating of .
Select problem
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 25, Problem 1 (PE) calculator screenshot 1

Comments

This video was updated on Jan. 16th, 2024.