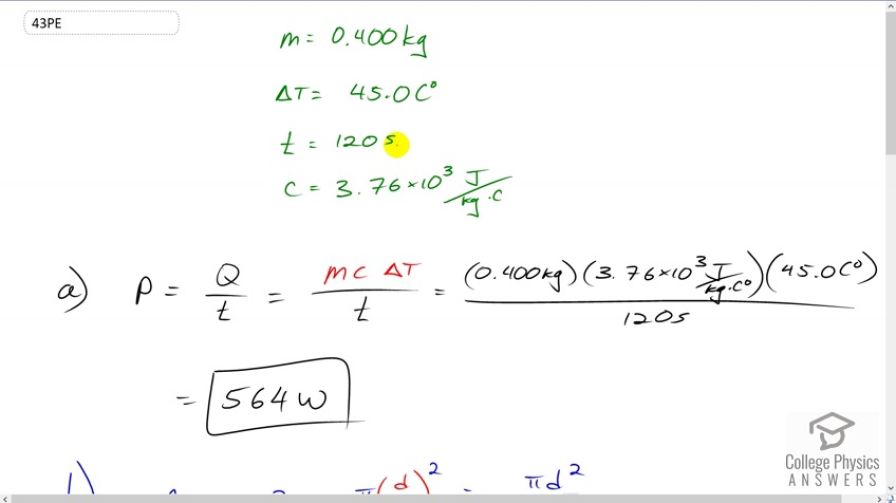
Question
On its highest power setting, a microwave oven increases the temperature of 0.400 kg of spaghetti by in 120 s. (a) What was the rate of power absorption by the spaghetti, given that its specific heat is ? (b) Find the average intensity of the microwaves, given that they are absorbed over a circular area 20.0 cm in diameter. (c) What is the peak electric field strength of the microwave? (d) What is its peak magnetic field strength?
Final Answer
Solution video
OpenStax College Physics, Chapter 24, Problem 43 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. 0.4 kilograms of spaghetti has its temperature increased by forty five Celsius degree within 120 seconds by this microwave and the specific heat of spaghetti we are told is 3.76 times ten to the three joules per kilogram per Celsius degree. Now the question is what is the power of the microwave? And so that's going to be the rate at which heat is transferred to the spaghetti. So that is Q is the amount of heat the spaghetti absorbs and divided by the time which we are given 120 seconds and the heat that it absorbs is the mass times specific heat times change in temperature. So that's 0.4 kilograms times 3.76 times ten to the three joules per kilogram per Celsius degree times 45 Celsius degrees all divided by 120 seconds gives the power of 564 Watts. The next question asks for the intensity of the microwaves and so we take the power and have to divide it by the area over which the power is absorbed and we're told that it's absorbed over a circular area with a given diameter. And so we'll find the area of that Circle which is Pie times radius squared but since we're given diameter we’ll substitute diameter divided by two in place of r and that means our area is Pie times diameter squared over four. So we'll substitute that in for area and I'm multiplying by its reciprocal instead of dividing by a fraction. So that's why you have the four on the top here. So four times power divided by Pie times diameter squared. So it's four times 564 Watts with what we calculated in part A divided by Pie times 20 centimeters written as times ten to the minus two meters and square that giving an intensity of 1.80 times ten to the four watts per square meter. The next question asks for the strength of the electric field in this electromagnetic wave and the intensity is speed of light times permittivity of free space times the electric field strength at its peak squared over two. And so we'll solve for E subscript oh E naught and we'll do that by multiplying both sides by two over C Epsilon naught and then we'll take the square root of both sides. And so we have the electric field at its peak equals the square root of two times the intensity divided by speed of light times permittivity of free space. So it's square root of two times 1.7953 times ten to the four watts per square meter that we calculated in Part b divide that by the speed of light times permittivity of free space and we get 3680 Newton's per coulomb is the peak electric field strength. Then in part d, we are asked for the magnetic field strength, and there's two ways we could have done it. This is one way and then the other way would use the average intensity formula and so on and so on but this is the simpler way to do it. So we'll multiply both sides by B naught over c . And then we solve for, oops, and then we solve for B naught . So the magnetic field strength at its peak is the electric field strength at its peak divided by speed of light. So the 3676.66 Newton's per coulomb we're using the unrounded version of this in order to avoid intermediate rounding error, and we divide that by three times ten to the eight meters per second giving 1.23 times ten to the minus Five Tesla.

