Question
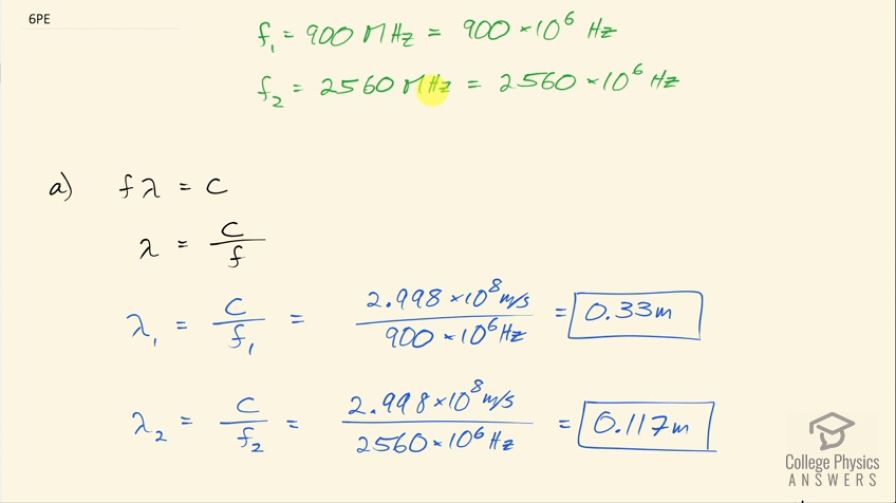
(a) Two microwave frequencies are authorized for use in microwave ovens: 900 and 2560 MHz. Calculate the wavelength of each. (b) Which frequency would produce smaller hot spots in foods due to interference effects?
Final Answer
- ,
- The frequency with the smallest wavelength will produce the smallest spots:
Solution video
OpenStax College Physics, Chapter 24, Problem 6 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The two frequencies that microwaves can use are 900 megahertz and 2560 megahertz which I have written with their times 10 to the 6 in place of the 'mega' prefix. So we want to know what is the wavelength of each of these frequencies because the size of the hotspot in part (b) will be proportional to the size of the wave. So the wave equation is that the frequency multiplied by its wavelength equals the speed of the wave and since we are talking about electromagnetic waves, we have a speed of c, which is the speed of light and we can solve for λ by dividing both sides by frequency. So the wavelength then is the speed of light divided by its frequency. So λ 1 is the speed of light divided by frequency one and that is 2.998 times 10 to the 8 meters per second divided by 900 times 10 to the 6 hertz, which is 0.33 meters. Wavelength number two is speed of light divided by frequency two and that works out to 0.117 meters. The frequency of the smallest wavelength will produce the smallest spot and that is frequency two— 2650 megahertz—since it has the shortest wavelength.
