Question
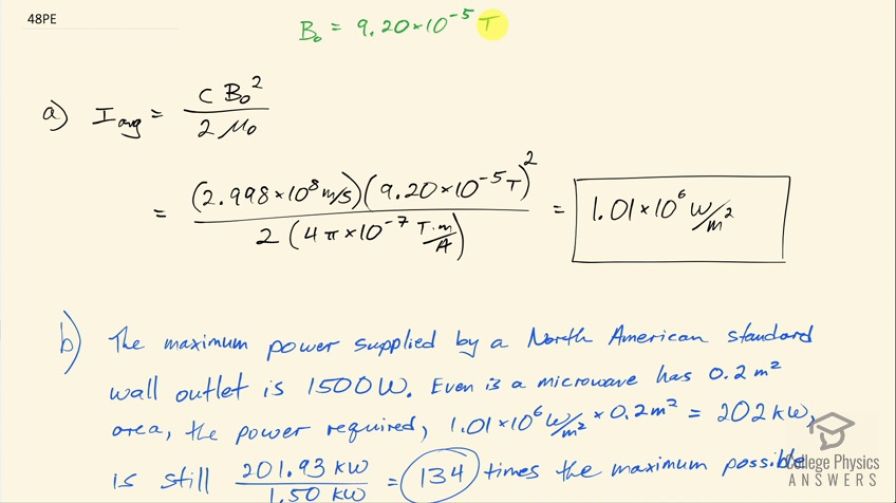
The peak magnetic field strength in a residential microwave oven is . (a) What is the intensity of the microwave? (b) What is unreasonable about this result? (c) What is wrong about the premise?
Final Answer
- The maximum power supplied by a North American standard wall outlet is 1500 Watts. Even if a microwave has an areas of only , the power required is still 134 times the maximum possible supply from the outlet. The intensity is too high.
- The stated peak magnetic field strength is too high.
Solution video
OpenStax College Physics, Chapter 24, Problem 48 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are told that a household microwave oven has a peak magnetic field strength of 9.20 times 10 to the minus 5 tesla and part (a) is asking us to calculate the intensity of this microwave. So that's going to be the speed of light times the peak magnetic field strength squared divided by 2 times the permeability of free space. So we have 2.998 times 10 to the 8 meters per second times 9.20 times 10 to the minus 5 tesla squared divided by 2 times 4π times 10 to the minus 7 tesla meters per amp and that's 1.01 times 10 to the 6 watts per square meter. This is too high; the maximum power that can be supplied by a North American standard wall outlet is 1500 watts... beyond that the circuit breakers will trip. So even if a microwave has a much smaller area than a square meter... let's suppose it has 0.2 square meters inside the microwave, the power required would be this intensity multiplied by 0.2 square meters, which is about 202 kilowatts which is still 134 times the power that's possible from a regular wall outlet. So the stated peak magnetic field strength is too high.
