Question
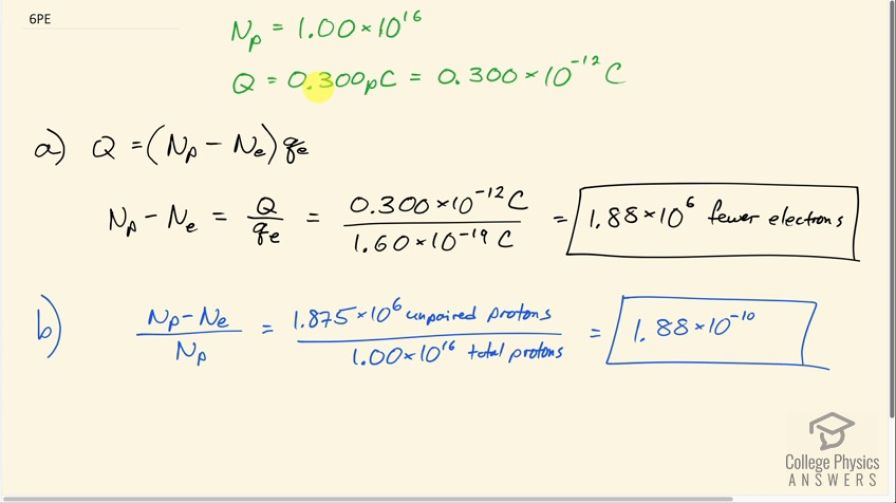
An amoeba has protons and a net charge of
0.300 pC. (a) How many fewer electrons are there than protons? (b) If you paired them up, what fraction of the protons would have no electrons?
Final Answer
- fewer electrons
Solution video
OpenStax College Physics for AP® Courses, Chapter 18, Problem 6 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An amoeba consists of 1.00 times 10 to the 16 protons and the net charge on the amoeba is 0.300 picocoulomb and the prefix 'pico' means times 10 to the minus 12 and question (a) asks us how many fewer electrons are there than protons or you could also ask... how many more protons are there than electrons— that would be the same thing— and so here we have the charge Q equals the number by which the number of protons exceeds the number of electrons multiplied by the elementary charge. So each unpaired proton gets multiplied by the elementary charge and that product makes the total net charge Q. So we can divide both sides by this elementary charge and you get the difference between the number of protons and electrons is the charge divided by elementary charge. So that's 0.300 times 10 to the minus 12 coulombs divided by 1.60 times 10 to the minus 19 coulombs which is 1.88 times 10 to the 6 extra protons or fewer electrons, whichever way you wanna say it. Part (b) asks us what fraction of the protons are unpaired with an electron? So we take this number here that we found in part (a)— that's the number of unpaired protons or protons that are additional to all the electrons— and we divide that by the total number of protons and this is 1.88 times 10 to the minus 10 is the fraction of protons that are unpaired.