Question
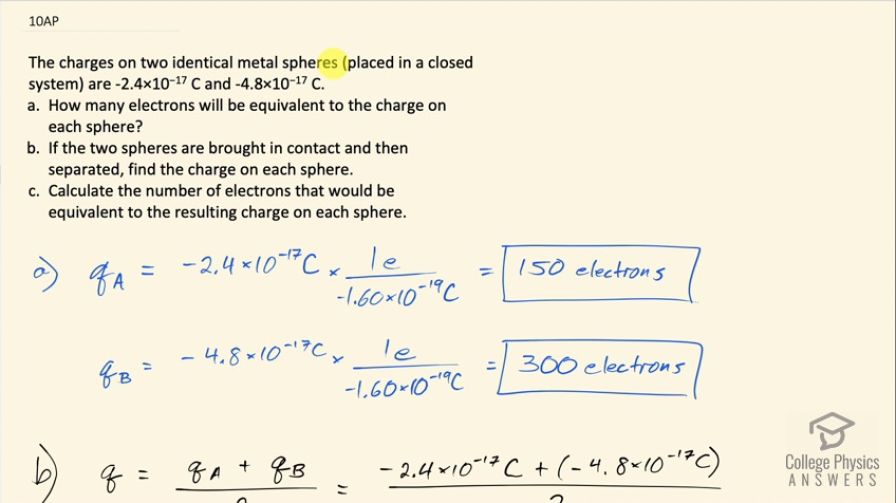
The charges on two identical metal spheres (placed in a
closed system) are and .
- How many electrons will be equivalent to the charge on each sphere?
- If the two spheres are brought in contact and then separated, find the charge on each sphere.
- Calculate the number of electrons that would be equivalent to the resulting charge on each sphere.
Final Answer
- 150 electrons and 300 electrons.
- 225 electrons.
Solution video
OpenStax College Physics for AP® Courses, Chapter 18, Problem 10 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. There are two identical metal spheres one has a charge of negative 2.4 times 10 to the minus 17 coulombs and the other has a charge of negative 4.8 times 10 to the minus 17 coulombs and in part (a), we are meant to figure out how many electrons is equivalent to the charge on each sphere. So let's call the first sphere—sphere A— and it has this charge negative— 2.4 times 10 to the minus 17— and we'll multiply that by one electron for every negative 1.60 times 10 to the minus 19 coulombs— this is the elementary charge with the charge on a single electron— and this works out to 150 electrons and then multiply the charge on the other metal sphere by the same fraction and we get 300 electrons. After the two spheres are brought in contact with each other, the charge will be evenly distributed among both spheres which means the charge on a single sphere after contact will be the average of the two charges initially. So we have negative 2.4 times 10 to the minus 17 coulombs plus negative 4.8 times 10 to the minus 17 coulombs divided by 2 and that's negative 3.6 times 10 to the minus 17 coulombs. And part (c) asks how many electrons would this new charge be equivalent to? So we multiply the answer for part (b) by one electron for every 1.60 times 10 to the minus 19 coulombs and that is 225 electrons. And notice that the total number of electrons in both cases before contact and after contact is the same. So before we had a total charge of 300 plus 150 for a total of 450 electrons whereas afterwards, we have 225 electrons on each sphere so times that by 2 also gives a net charge of 450 and the net charge has to remain constant due to the conservation of charge.

