Question
(a) By what factor must you change the distance between two point charges to change the force between them by a factor of 10? (b) Explain how the distance can either increase or decrease by this factor and still cause a factor of 10 change in the force.
Final Answer
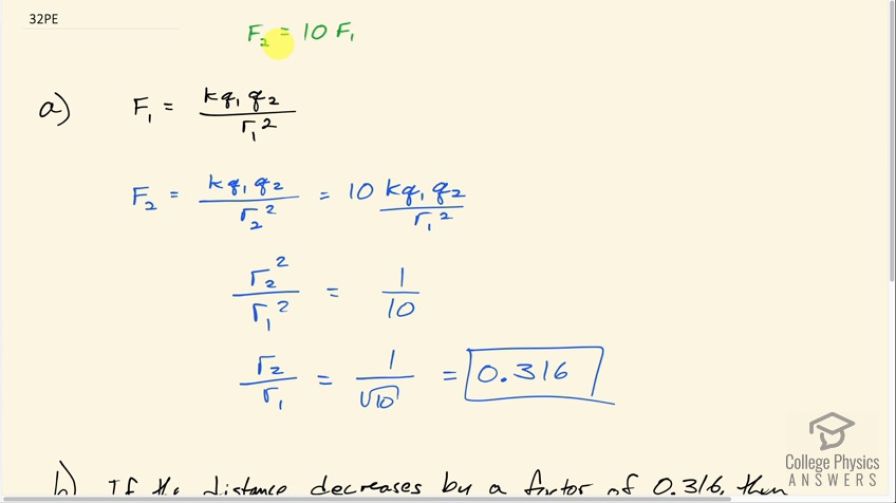
- The distance must be reduced by a factor of 0.316 in order to increase by a factor of 10.
- If the distance decreases by a factor of 0.316, then the force will increase by a factor of 10. If the distance increases by a factor of 3.16, then the force will decrease by a factor of 0.1. The wording of the question leaves room for interpretation about what changing by a "factor" means. When I use the word factor, I intend it to mean a number that multiplies.
Solution video
OpenStax College Physics for AP® Courses, Chapter 18, Problem 32 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We want to know by what factor we have to change the distance between two point charges such that the force between them changes by a factor of 10. So the wording leaves some room for interpretation and I am gonna assume that they mean that force 2 is 10 times force 1 because factors multiply... at least that's my interpretation of the word 'factors' because when you try to factorize the number 6, say you find two numbers that multiply to make it and these two numbers are called factors so factors multiply so changing the force by a factor of 10 means that the new force will be 10 times the old force. So the first force is Coulomb's constant times some charge 1 and times some charge 2 divided by some distance r 1 squared and F 2 will be kq 1q 2—same charges— divided by some new distance, r 2 squared, and we want to find by what factor these distances are different? So we can divide both sides by kq1 q2 so they cancel on both sides and then we are gonna multiply both sides by r squared... r 2 squared I should say... and also divide both sides by 10. So in the end, we are left with 1 over 10 on one side and then we are left with r 2 squared over r 1 squared on the other side. So we take the square root of both these sides and we get r 2 over r 1 then is 1 over the square root of 10 which is 0.316. So the new distance r 2 is different from r 1 by a factor of 0.316—it's been reduced by a factor of 0.316. Okay! And then in part (b), it says explain how the distance can either increase or decrease by this factor and still cause a factor of 10 change in the force and the wording here brings a debate about what it means to change something by a factor. So I am gonna pick a side on the debate and say that factors always multiply. So when you say that distance decreases by a factor of 0.316, it's to say that the distance is now 0.316 what it was before and by multiplying by a number less than 1, you are reducing it. And then the force will increase by a factor of 10 which is to say the new force will be 10 times the old force or if the distance increases by a factor of 1 over 0.316 which is 3.16 so I'm multiplying by a number that's greater than 1 now then the force will decrease by a factor of one-tenth which is 0.1 and the wording that the textbook seems to suggest is when you know you have a force that decreases by a factor of 10 and decreasing means divide by the factor but that's kind of confusing because to me the word 'factor' means multiply so if you are gonna decrease by a factor then you must have a factor that is less than 1 as we do here by going 1 over 10 which is 0.1. Okay... there we go!
