Question
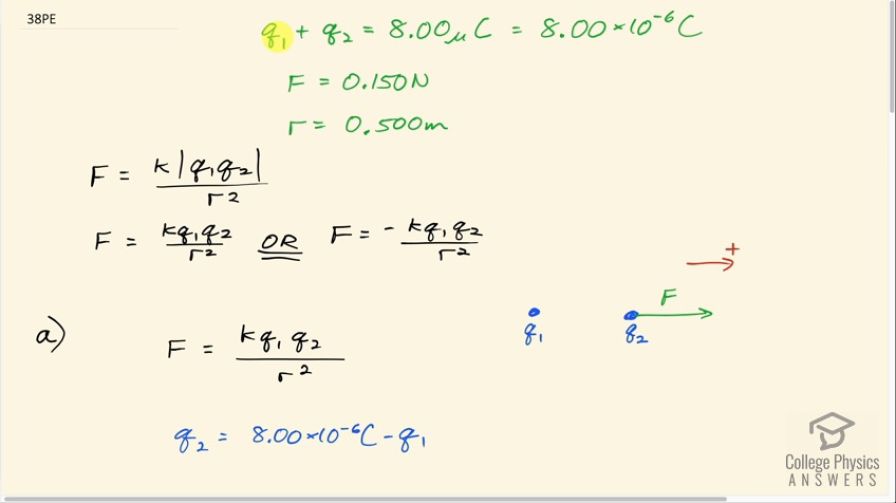
(a) Two point charges totaling exert a repulsive
force of 0.150 N on one another when separated by 0.500 m. What is the charge on each? (b) What is the charge on each if the force is attractive?
Final Answer
- One charge is whereas the other is
- One charge is whereas the other is
Solution video
OpenStax College Physics for AP® Courses, Chapter 18, Problem 38 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Two charges add up to 8.00 microcoulombs; we are not told what the charges are and that's going to be the question to answer what is q 1 and what is q 2. We are just told if you were to add the charges together, here's what their sum would be. The force between them is 0.150 newtons and in part (a) that's a repulsive force and the distance between them is 0.500 meters. So Coulomb's Law says that the electrostatic force is Coulomb's constant times the absolute value of the product of the charges divided by the distance between them squared. So when you are solving an absolute value equation, you don't know whether this stuff in between the vertical lines is positive or negative and so when solving it, you are going to get two answers: one where you assume it's positive; and the second where you assume it's negative. And so this is the force: positive kq 1q 2 over r squared or the force is negative kq 1q 2 over r squared and in part (a), we'll take the assumption that it's positive which is this scenario here where we have the two charges arranged this way where 'to the right' is positive and the force is repulsive and so it will be positive to the right and this is for the assumption that the force is repulsive and the charges are the same. Okay! And then in part (b), we'll say that the force is negative in which case the charges are different and notice that the number that you substitute in for q 1 and q 2 are always magnitudes and so you have to introduce this negative sign into the formula to account for their different signs. Okay! So q 2 is 8.00 times 10 to the minus 6 coulombs minus q 1 after rearranging this here to solve for q 2 and then we can make a substitution for q 2 and replace it with 8.00 minus q 1. And then we'll multiply both sides by r squared and you have r squared times the force equals the sum times kq 1 minus kq 1 squared when you multiply this kq 1 by both terms in the brackets and then we'll bring this term to the other side and so we'll add kq 1 squared to both sides and then we'll take this term to the other side as well by subtracting it from both sides and we end up with this line which is a quadratic equation and we'll use our quadratic formula to solve it. And let's first divide everything by k, which we will do in the next step here actually so we'll divide everything by this 8.988 times 10 to the 9 so that it just cleans up the coefficients for these first two terms and makes them a bit simpler to write. So we end up with this line here where we have 1 times q 1 squared minus 8.00 times 10 to the minus 6 q 1 plus this 0.500 meter separation squared times the force of 0.150 newtons divided by 8.988 times 10 to the 9 all this equals zero and we will figure out what this constant term is by you know calculating and it works out to 4.17223 times 10 to the minus 12; I am not gonna bother writing in units anymore because this is a lot of writing and the units are a bit strange anyway. So the quadratic formula says that the variable... the thing that's squared is the negative of the linear coefficient plus or minus the square root of the linear coefficient linear meaning the coefficient of the term to the power of 1 minus 4 times the coefficient of the quadratic term multiplied by the constant term all divided by 2 times a. So a is 1, b is this negative 8 times 10 to the minus 6 and then c is this thing here so that's c, this is b and this is a so then we plug it in down here. So b is negative 8 times 10 to the minus 6 and the formula says take the negative of whatever b is and so negative and a negative makes positive 8 times 10 to the minus 6 plus or minus the square root of 8 times 10 to the minus 6 squared minus 4 times 1 times 4.17223 times 10 to the minus 12 all divided by 2. This works out to 7.44 microcoulombs and 0.561 microcoulombs depending on whether you take the plus or the minus there. And we could have answered the question a slightly different way by taking just the plus suppose and you get this answer and then take the q 2 as 8 minus that answer and they would end up with 0.561 just the same. Okay! So that's part (a) and then part (b) we say that the force is pointing in the other direction— it's negative 0.150 newtons— and when doing that that's the only change... when doing that that makes this term here this constant term become negative because we put in negative 0.150 in there so we'll just put a minus in front of this and otherwise everything else is the same. So we are gonna be solving the quadratic equation where we have q 1 squared minus 8 times 10 to the minus 6q 1 minus 4.17223 times 10 to the minus 12 and plugging that into the quadratic formula gives negative 4.91 times 10 to the minus 7 and 8.49 times 10 to the minus 6. So we can say that one charge is 8.49 microcoulombs whereas the other one is negative 0.491 microcoulombs and you will find that if you add these two numbers together, you will end up with 8 microcoulombs as we expect.



