OpenStax College Physics, Chapter 30, Problem 7 (Problems & Exercises)

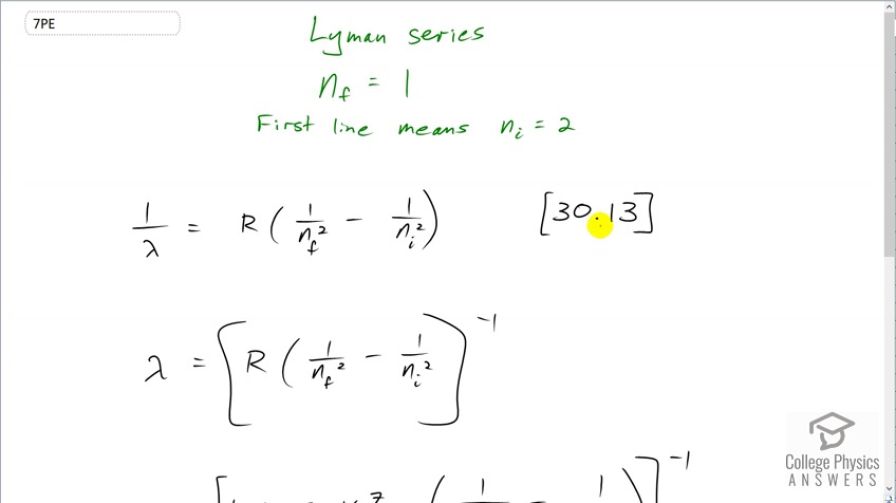
This is College Physics Answers with Shaun Dychko. This formula tells us what the wavelength is in the spectrum for a hydrogen-like atom; it's equation [30.13]. And it says that the reciprocal of the wavelength in the spectrum is Rydberg's constant times 1 over the final energy level squared minus the 1 over the initial energy level squared. The Lyman series means that the final energy level is 1 which is the minimum energy level, the ground state, in other words. And this initial energy level has to be higher than this one in order to have a transition down to it and so the first line is gonna have an initial equal to 2. And so we solve this for λ by taking the reciprocal of both sides which means raise both sides to the power of negative 1. And we could do some fancy algebra here to turn this into a fraction by having a common denominator and then flipping it after we take the reciprocal but I prefer to just wrap the whole thing in brackets and make it exponent negative 1. So on the left side we did flip and it's now λ over 1 although we don't write that denominator 1 of course. So it's λ equals R times 1 over n f squared minus 1 over n i squared and take the whole thing to the power of negative 1. So we have 1.097 times 10 to the 7 reciprocal meters— that's Rydberg's constant— times 1 over the final energy level squared minus 1 over the initial energy level which is 2, squared and then take that whole result to the power of negative 1 and we get 122 nanometers is the wavelength. And 380 nanometers is the shortest wavelength that is visible. So that means this wavelength is shorter than that which makes it ultraviolet.