Question
(a) Which line in the Balmer series is the first one in the UV part of the spectrum?
(b) How many Balmer series lines are in the visible part of the spectrum?
(c) How many are in the UV?
Final Answer
- . This is the 8th line.
- There are 7 visible lines in the Balmer series.
- There are an infinite number of lines in the Balmer series in the ultra violet part of the spectrum.
Solution video
OpenStax College Physics, Chapter 30, Problem 18 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
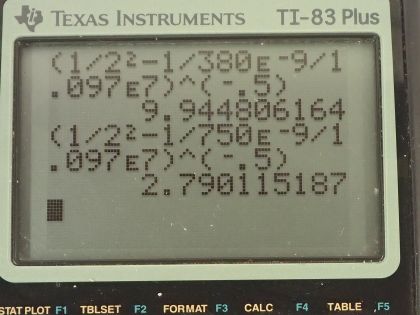
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We want to know which line in the Balmer series is the first one in the ultraviolet part of the spectrum? So the Balmer series ends with a principal quantum number of 2 and so n f is 2 in this formula which tells us the reciprocal of the wavelength emitted is Rydberg's constant times 1 over the final principal quantum number squared minus 1 over the initial principal quantum number squared; let's just call this the orbital number— that's a little easier to say. So we are going to set the wavelength equal to 380 nanometers because anything less than that is going to be ultraviolet and so the very first one will occur at 380 nanometers. So we are going to divide both sides by R and we get 1 over n f squared minus 1 over n i squared equals 1 over λ times Rydberg's constant and then add 1 over n i squared to both sides and subtract 1 over λR from both sides and then you get this line here after you switch the sides around so 1 over n i squared equals 1 over n f squared minus 1 over λR. If you raise both sides to the exponent negative one-half the negative sign flips this fraction to get n i on the top and the one-half takes the square root of it so exponent negative one-half turns 1 over n i squared into n i and we have to do the same thing to the other side. So we have the initial orbital number is going to be 1 over the final orbital number squared minus 1 over the wavelength times Rydberg's constant all to the negative one-half. So that's 1 over 2 squared minus 1 over 380 times 10 to the minus 9 meters times Rydberg's constant all to the one-half which is 9.9448. Now because the principal quantum number is quantized, it can have only discreet whole numbers are possibilities for it so you can't have a decimal so n i then we'll take to be 10, we'll take the first number greater than this that will work and that is 10 and it's the 8th line in the series because if you end at 2 you know and you start at 10, 10 minus 2 is 8 and there we go! Then in part (b), how many Balmer series lines are in the visible part of the spectrum? So we want to know how many are in between the wavelengths of 380 nanometers up to 750 nanometers? So we have already found the initial orbital number for 380 nanometers—it's 10— although we'll take it to be 9 in this case because we want the first number less than this in order for it to be visible because greater than 9.9448 will result in a higher energy and therefore shorter wavelength pushing it below 380 nanometers which is into the ultraviolet and the first number less than 9.9448 will be 9 and it will be with an energy that's reduced and therefore has a bigger wavelength so that's in the visible part of the spectrum. So n i prime then— prime meaning for this second scenario of 750 nanometers— is 1 over 2 squared repurposing this formula here by the way— minus 1 over 750 times 10 to the minus 9 meters times Rydberg's constant all to the negative 1 is 2.79 and we'll take this to be 3 because if it was 2 then the energy would be less and the wavelength therefore longer than the number we have chosen and we don't want a wavelength longer than 750— that would not be visible anymore— so we take n initial prime is 3. So all of the initial orbital numbers between 3 and 9 inclusive will work and that is a total of 7 possible lines in the Balmer series that are visible. So when you count between 3 and 9 9 minus 3 is 6 but we are inclusive so we are including 3 and a 9 and so you add 1 to that difference and the answer is 7. Okay! Part (c) is how many are in the ultraviolet part of the spectrum? So ultraviolet goes from 10 nanometers up to 380 nanometers; we already know the initial orbital number for 380, let's find out what it should be for 10 nanometers? So that's 1 over 2 squared minus 1 over 10 times 10 to the minus 9 meters times Rydberg's constant all to the negative one-half but we will find that this difference is negative and so we can't take the square root of that negative—it's undefined— which we interpret to say that n i prime then is infinite. So every single transition that goes from n i equals 10 as its initial starting point to an n i is 11 or starts at 12 or is 13 or 14 and so on it goes all the way up to infinity there's a number of possibilities that are here for states to start in and all of them will end up being ultraviolet everything from infinity to 10 and the number of possibilities then is infinite. So there are an infinite number of lines in the Balmer series in the ultraviolet part of the spectrum.