OpenStax College Physics, Chapter 30, Problem 19 (Problems & Exercises)

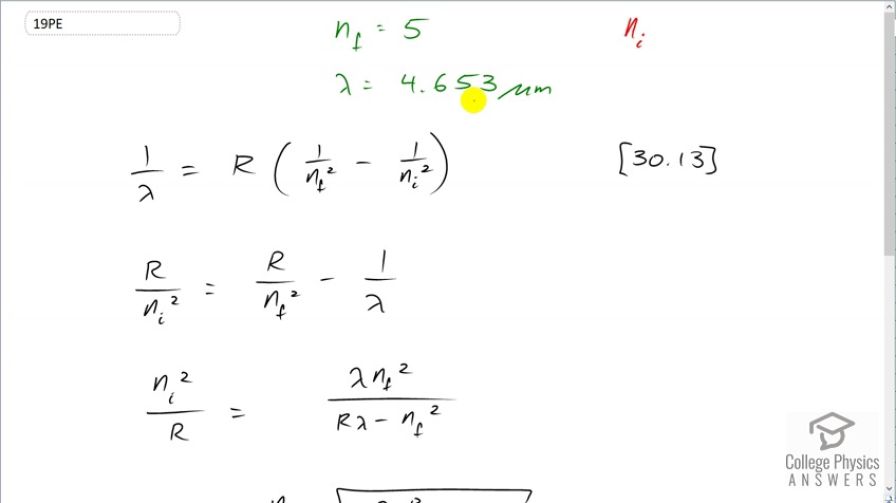
This is College Physics Answers with Shaun Dychko. A hydrogen atom emits a wavelength of 4.653 micrometers when an electron transitions from some initial state that we don't know to a final state of 5. Now equation [30.13] tells us what the wavelength is that's emitted as a function of the final and initial states of the electron and we need to solve this for n i. So let's first multiply through by Rydberg's constant and then get the R over n i squared term on the left side and that's gonna give us R over n i squared equals R over n f squared minus 1 over λ. And then we'll take the reciprocal of both sides but let's also get a common denominator here; we'll multiply top and bottom of this by λ and top of bottom of this by n f squared over n f squared and this makes Rλ minus n f squared all over λn f squared. And then we'll take the reciprocal of both sides which means flip these two fractions so that we get our unknown n i in the numerator. So it's n i squared at the moment divided by R and that equals λn f squared over Rλ minus n f squared. And then multiply both sides by R and then take the square root of both sides as well and we end up with n f to the power of 1 which because it's squared we can take it out of the square root sign and call it n f to the power of 1 times square root of λ times Rydberg's constant divided by Rλ minus n f squared. And so we have the final energy level we are told is 5 and we'll multiply that by the square root of 4.653 times 10 to the minus 6 meters times Rydberg's constant divided by the Rydberg's constant times the wavelength of the emitted photon minus n f squared so that's 5 squared and this works out to an initial energy level of 7.