Question
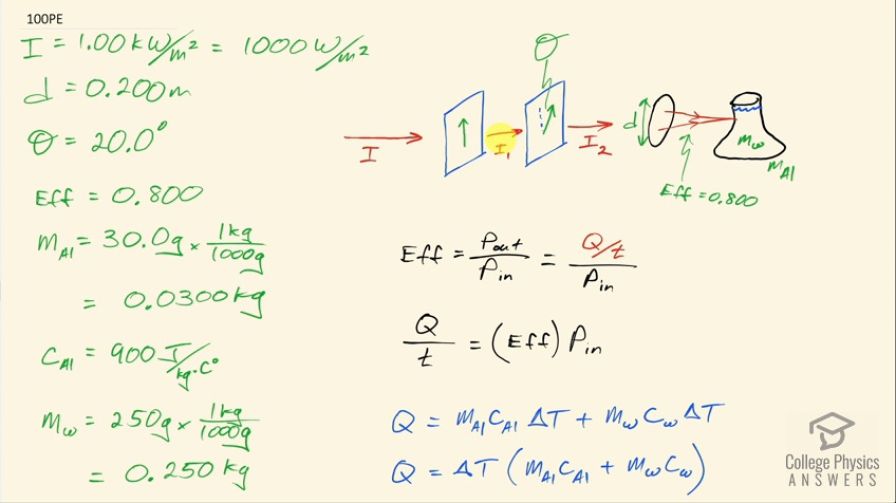
(a) On a day when the intensity of sunlight is , a circular lens 0.200 m in diameter focuses light onto water in a black beaker. Two polarizing sheets of plastic are placed in front of the lens with their axes at an angle of . Assuming the sunlight is unpolarized and the polarizers are 100% efficient, what is the initial rate of heating of the water in , assuming it is 80.0% absorbed? The aluminum
beaker has a mass of 30.0 grams and contains 250 grams of water. (b) Do the polarizing filters get hot? Explain.
Final Answer
- Since the polarizers are 100% efficient, they don't absorb any of the sunlight's energy. Therefore they do not get hot.
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 100 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Unpolarized sunlight is going to be hitting a polarizing film, passing through it and then encountering another polarizing film with its polarization axis at an angle Θ with respect to the polarization axis of the first film and then after passing through that the sunlight will go through a lens that has a diameter of 0.200 meters and get focused onto a beaker that is made out of aluminum and has a mass of 30.0 grams and this beaker is full of some water— 250 grams of it— and this water and beaker are going to be heated up by the sunlight and the question is what is the rate of temperature increase of the water-beaker system? So we are also told that there is a certain efficiency of absorption of the sunlight and that efficiency is 80 percent so that efficiency is the power that is absorbed by the water and aluminum divided by the power that's input from the Sun. So you have to fasten your seat belt here— it's a long solution— it's interesting because it ties in a lot of different concepts let's run through the information given to us first of all: I have already talked about the diameter of the lens; we have the intensity of sunlight is 1.00 kilowatt per square meter, which is a 1000 watts per square meter; we have this efficiency of absorption of the sunlight what else do we need to have... we have the specific heat of aluminum, which is 900 joules per kilogram per Celsius degree— we looked that up in table [14.1]— and we also need to find the specific heat of water— it's 4186 joules per kilogram per Celsius degree— and we assume that this water is at 15.0 degrees Celsius because the question doesn't tell us otherwise so we have written that piece of information here but we have converted the masses into kilograms by multiplying by 1 kilogram for every 1000 grams so we have 0.0300 kilograms of aluminum and 0.250 kilograms of water. Okay! So let's get to work! Efficiency is power absorbed divided by power delivered by the Sun, the power absorbed is going to be the heat absorbed divided by time so this is the rate of heat absorption and the amount of heat absorbed—Q— we have an expression for that but first let's solve for Q over t by multiplying both sides by power input. So the rate of heat absorption is the efficiency times the power delivered by the Sun. The heat absorbed is going to be the mass of the aluminum times the specific heat of aluminum times its change in temperature plus the mass of the water times the specific heat of water times its change in temperature but we assume that the change in temperature of the aluminum and water is the same because they are in contact with each other so we'll factor out this ΔT then and then substitute all of this in place of Q here. So we have change in temperature times mass of aluminum times specific heat of aluminum plus mass of water times specific heat of water divided by time is this efficiency times the power input. And we can solve for ΔT over time by dividing both sides by this bracket and we get this expression here so now we have an expression for the rate of increase in the temperature and that's what we want to find but we need to work on this right-hand side a little bit to express this power input in terms of the things that we know. So the power input is going to be the intensity of the sunlight here multiplied by the area of this lens. So intensity is the amount of power—watts— per area—meter squared— and so we need to express this lens area in meters squared and because it's going to be all of the power delivered over this area of the lens that's going to be given to the beaker in water, you know, keeping in mind this efficiency of 80 percent. So the power input then is going to be the intensity that's passed through the second polarizing film multiplied by the area of the focusing lens so that lens area is going to be π times its radius squared but we are given the diameter so we'll substitute diameter divided by 2 in place of the radius and we end up with I 2 times π times diameter squared over 4. So this gets substituted in for the power input and I write that here in red so I have I 2πd squared over 4 written in place of power input. Then we need to figure out what is this I 2? Well I 2 is going to be I 1 times cos squared Θ— this is the formula for the intensity given an angle Θ between the axis of polarization of a polarizing film and the direction of polarization of the incident light. So the light traveling between these polarizing films will have a polarization upwards since it just passed through this polarizing film with its polarization upwards and so I 2 is going to be I 1 multiplied by cos of this angle Θ squared and so that's what we say here but now what is I 1 compared to I naught? This is the intensity given to us— the intensity of the Sun— and I 1 is going to be half of the intensity of the Sun because unpolarized light passing through any polarizing film will have its intensity reduced by half because on average, you have a scattering of different polarization directions of all this light; this light is a mixture of different directions evenly distributed among all the directions so on average half of it is upwards and then half of it is across. So all the polarizations that are across will get filtered out and the polarizations that are upwards will pass through. So I 1 is going to be half of I naught so we substitute that in place of I 1. So I 2 then is I naught over 2 times cos squared Θ so we substitute that in here and that's shown here in red. So at long last, we have a final formula for the rate of temperature increase and that's going to be the efficiency times I naught times π times d squared times cos squared Θ divided by 8, which is this 4 times this 2 here because we can multiply top and bottom by 2 and this 2 cancels with this 2 and we are left with 2 times 4 on the bottom and then times this bracket. So we have 0.800—efficiency— times a 1000 watts per square meter— intensity originally from the Sun— times π times the 0.200 meter— diameter of the lens—squared times cos of 20.0 degrees and then square that divided by 8 times 0.0300 kilograms— mass of aluminum— times 900 joules per kilogram per Celsius degree— specific heat of aluminum— plus 0.250 kilograms—mass of water— times 4186 joules per kilogram per Celsius degree and this is the rate of temperature increase then of 0.0103 Celsius degrees per second. Alright! If you have made it this far, give yourself a pat on the back that was a long solution to get through and hopefully it made sense. Part (b) says will the polarizing filters get hot? Well, the question told us the polarizers are 100 percent efficient so that means they don't absorb any of the sunlight's energy and that means they do not get hot.
