OpenStax College Physics for AP® Courses, Chapter 27, Problem 8 (Test Prep for AP® Courses)

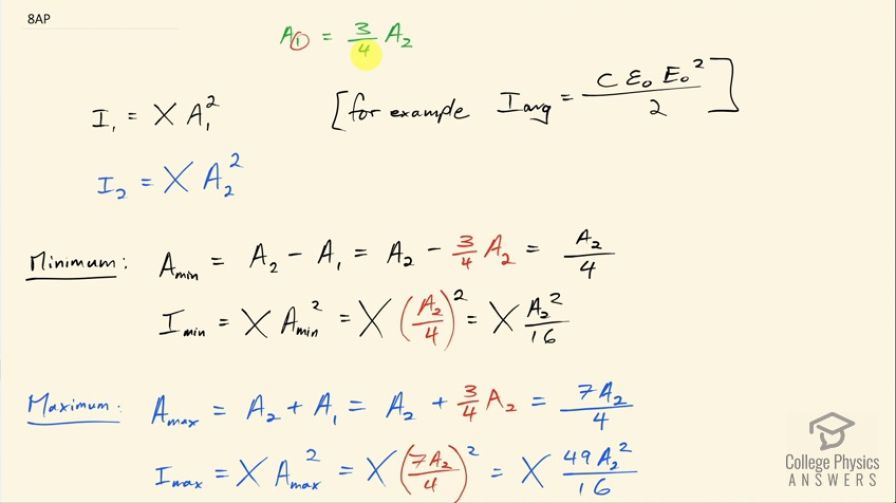
This is College Physics Answers with Shaun Dychko. In a double slit experiment, we have the amplitude of the wave going through one of the slits is three quarters the amplitude of the wave going through the second slit— that's what it means to say that they have a three to four ratio of their amplitudes. The intensity of the first wave is some constant multiplied by the square of the amplitude and if we were talking about the electric field strength in this electromagnetic wave, we could say that the average intensity is speed of light times permittivity of free space times the peak electric field squared divided by 2— that's one of our formulas— we also have another formula for the average intensity in terms of the magnetic field strength in the electromagnetic wave but it doesn't really matter which one we are talking about, this one just says amplitude in general so one or the other in either case, the intensity is proportional to the amplitude squared. And the intensity of the second wave will be the same constant if we were talking about electric field, the constant would be speed of light times permittivity of free space over 2 but again not important, what's important is to say that the intensity depends on the amplitude squared so intensity two is some constant times amplitude two squared. Okay! Now when there's a minimum in the interference pattern that means these two waves are perfectly out of phase and so one will be subtracting from the other so we'll have amplitude two minus amplitude one will be the net result that we'll see on the screen although what we'll see is the intensity actually... the brightness will be related to the intensity and not the amplitude and the difference is that intensity is dependent on the square of the amplitude. Okay! So amplitude one can be replaced with three quarters of amplitude two and we end up with amplitude two over 4 will be what we see at the minimum so the minimum will not be zero because the waves are of different amplitudes; if the waves are of the same amplitudes then subtracting one from the other would make zero but in this case we are subtracting only three quarters away from amplitude two. Okay so we are left with amplitude two over 4 is the minimum. The intensity there will be some constant times that minimum squared and that ends up being x times amplitude two squared over 16. At its maximum, we are going to be adding the two amplitudes and so we have A 2 plus three quarters of A 2 which is after you multiply this by 4 over 4 7A 2 over 4 and so the intensity at the maximum will be some constant times this maximum amplitude squared and that works out to 49A 2 squared over 16 and now we'll find the ratio of those intensities so we'll take the minimum intensity divided by the maximum intensity. So that's X times A 2 squared over 16 for the minimum intensity divided by this fraction but I am going to instead multiply by its reciprocal in order to keep everything on one line here so I am multiplying by this flipped over so times by 16 over X times 49A 2 squared, the A 2 squared's cancel and so do the X's and so do the 16's and we are left with 1 over 49. So that means the ratio of the minimum intensity to the maximum intensity is 1 to 49, or written as a ratio as 1:49.