OpenStax College Physics for AP® Courses, Chapter 27, Problem 3 (Problems & Exercises)

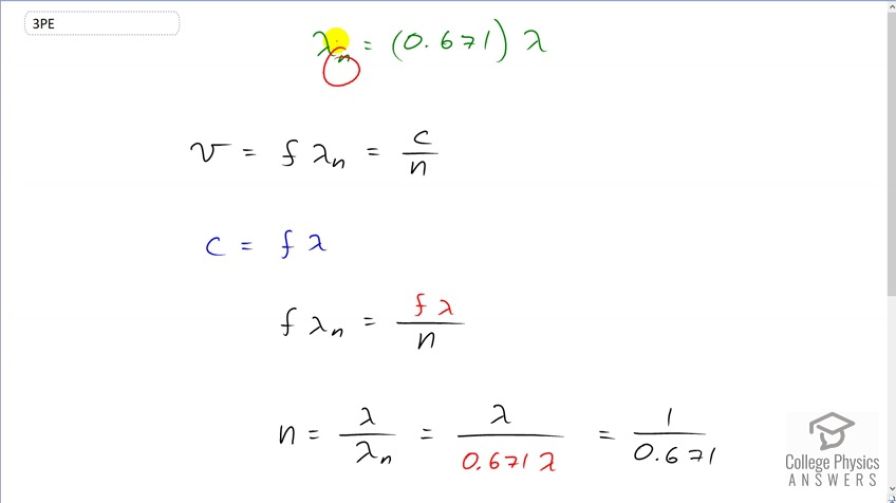
This is College Physics Answers with Shaun Dychko. We're told that the wavelength of some certain frequency of light in some medium that we don't know which has some index of refraction N is 0.671 times the wavelength of that same light in a vacuum. Now, the wave equation says that the speed of the wave is its frequency times its wavelength. And, the speed of the wave also is the speed of light in a vacuum divided by the index of refraction. And, the speed of light in a vacuum is also frequency times the wavelength in a vacuum. That's just using this wave equation in the case of a vacuum, in which case the speed would be the speed of light in a vacuum C. So, when we write frequency times the wavelength in the medium equals C over N, we can replace the C with frequency times Lambda, which I've done here. And then, solve this for N, we can multiply both sides by N over frequency and the frequency cancels. And then also, divide both sides by Lambda N. And, we get that the index of refraction is the wavelength in a vacuum divided by the wavelength in the medium. And, the wavelength of the medium we can substitute with this information we were given that it's the 0.671 times the wavelength in the vacuum. And now, these Lambdas cancel and we're left with the index of refraction is one over 0.671, which is 1.49. So, the index of refraction is 1.49 and looking up on the data table, this is polystyrene.