Question
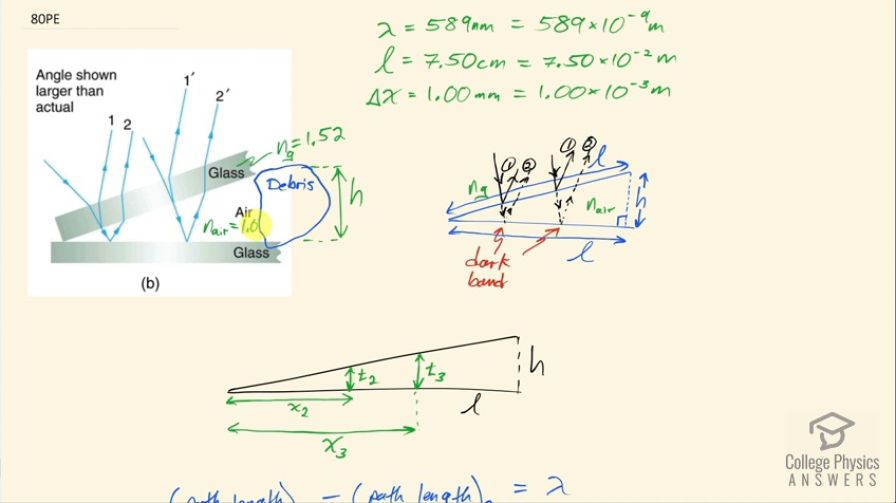
Figure 27.34 shows two 7.50-cm-long glass slides illuminated by pure 589-nm wavelength light incident perpendicularly. The top slide touches the bottom slide at one end and rests on some debris at the other end, forming a wedge of air. How thick is the debris, if the dark bands are 1.00 mm apart?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 80 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Two pieces of glass are separated by a small air gap here due to a piece of debris that's holding up one end and the other end of this glass slide is touching the other piece of glass. So there are some dark bands wherever there is destructive interference between the ray that's reflected from this glass-air interface versus the ray that's reflected here from the air-glass interface. So rays one and two will destructively interfere here and then they will destructively interfere again here and you see a dark band when your eyes are looking at it from above you are going to see a dark band here and you will see another dark band here and we are told that these dark bands are separated by a distance Δx, which is 1.00 millimeter. We are also told that the length of a glass slide is 7.50 centimeters and the wavelength of light that we're using is 589 nanometers. So you'd have to use a single wavelength of light in order to see this pattern because different wavelengths will have dark bands at different positions and they would all wash each other out so you wouldn't notice them but with a single wavelength, you will see these dark bands. Okay! So... I think probably we'll look at this picture here this picture is kind of reproducing this picture here where I have labeled some things on here where this is the height is the height of the debris and this distance l is the length of a glass slide. Now we are going to take this horizontal glass slide to be length l and we'll take this to be a 90 degree angle that's not strictly true because this hypotenuse will be a length l for sure and this leg here will be somewhat less than the hypotenuse but with a really small angle, the hypotenuse and this adjacent side will be pretty much the same, which is another way of saying that cosine of an angle that's small is pretty much 1 because cosine is the ratio of this adjacent to the hypotenuse and this is a y equals cos Θ graph and this is 1 when you have a zero angle and it's close to 1 when you have an angle close to zero. Okay! So that's a little approximation. Suppose we have a dark band here at some thickness of the air gap t 2 and it's going to occur at a position x 2 horizontally from the point of contact between the glass slides then we'll have another dark band at an air thickness of t 3 at a distance x 3 from the point of contact and these different thicknesses will cause different amount of path lengths traveled by the light and the path length at position 3 minus the path length at position two has to be a full wavelength because if there is going to be destructive interference here then... due to some path length difference then we are going to have destructive interference again when that path length difference is a full period more or in other words, a full wavelength more. Okay! So the path length at position 3 is 2 times this thickness of the air gap because light travels once down and then again up again so there's a round trip happening there so that's 2 times this air gap t 3 and then the path length at position 2 is 2 times whatever this thickness is and so we substitute in 2 times t 3 for the path length at position three and then two times t 2 substituted for path length at position 2 and all that equals the wavelength and then divide both sides by 2 and we get then that the difference in the air gap thickness has to be one-half of the wavelength. Okay! And that's as much as we can say when we're on the topic of air gap thickness. Now we can switch to thinking about the horizontal difference in these dark bands. So we are told that they occur every 1.00 millimeter so that means x 3 minus x 2 is going to be this Δx, which is 1.00 millimeter. Then we'll express x 3 and x 2 in terms of the height h, which is ultimately what we want to find, what is the height of this debris? So from similar triangles, we know that this horizontal distance x 3 divided by this vertical distance t 3 is equal to the horizontal distance l divided by this height h because the triangle in yellow is similar to the triangle in blue and when I say similar I mean that in a mathematically precise way this is not a colloquial, kind of looks the same it's to say that the angles in these two triangles are all equal and so given equal angles in these two triangles because they share an angle here and they are each a right triangle and so that must mean the third angle is the same as well so all the angles are the same in which case the ratio of corresponding sides is the same. So we take the horizontal leg divided by the height in each triangle and that ratio will be the same. So that's what I am saying here so x 3 divided by t 3 equals l over h in which case x 3 is l times t 3 over h so now we are expressing x 3 in terms of this h and that's good because we want h to appear in our formula because we want to solve for it. Same story for x 2 over t 2 it's also l over h in which case x 2 is lt 2 over h when you multiply both sides by t 2. Okay! Substituting for x 3 and x 2 in this then we have lt 3 over h minus lt 2 over h equals Δx and factoring out the l over h then gets multiplied by t 3 minus t 2 and that equals Δx and then times both sides by h over Δx and then switch the sides around to solve for h. So h is l times the difference in thicknesses of the air gap divided by Δx and we have an expression for this already t 3 minus t 2 is half the wavelength so we substitute that in and make it look nicer by putting this denominator 2 in denominator of the overall fraction we have lλ over 2Δx. So the length of a glass slide is 7.50 centimeters written as 7.50 times 10 to the minus 2 meters and we are using 589 nanometer light divided by 2 times 1.00 millimeter and that gives us 22.1 micrometers must be the size of the debris.
