Question
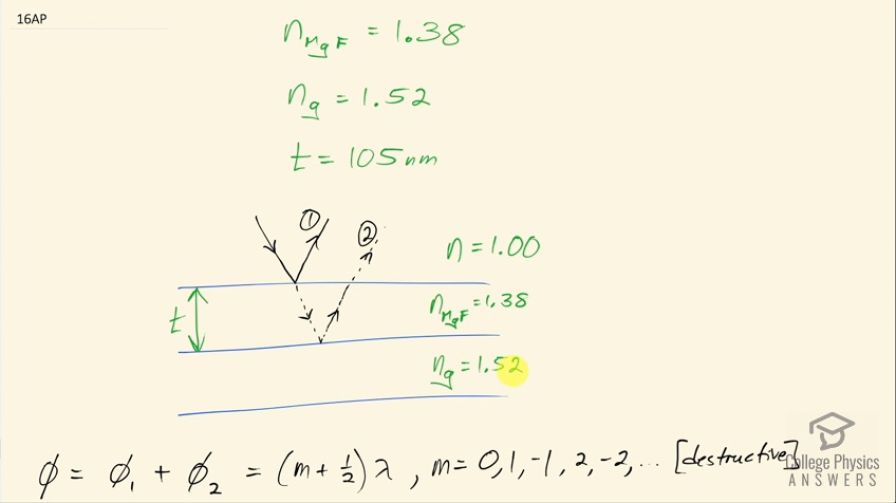
A film of magnesium fluoride (n = 1.38) is used to coat a glass camera lens (n = 1.52). If the thickness of the film is 105 nm, calculate the wavelength of visible light that will have the most limited reflection.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 16 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Some white light is hitting a camera lens that's made out of glass with index of refraction 1.52 but the lens is coated with a 105 nanometer thick layer of magnesium fluoride as an anti-reflection coating and the index of refraction of magnesium fluoride is 1.38 and the question is which color will be most strongly suppressed by this magnesium fluoride coating? So which color or which wavelength will experience the most destructive interference? So there's going to be a phase difference between this ray one and ray two ray one results from reflection from this air-magnesium fluoride interface and ray two travels through the magnesium fluoride and then reflects off of this interface between the magnesium fluoride and the glass and then travels back up through the thickness again and then since ray two travels at different distance than ray one, there will be a phase shift due to that increased path length that ray two travels and then in addition to that, there's also going to be a phase difference due to reflection from these interfaces. Now the way I have drawn it, I have this ray coming in at an angle and then reflecting off at this angle here but in reality, the ray's coming straight down and reflecting straight up but in order to distinguish between these two rays—ray one and ray two— I just drew it on an angle for convenience of making my drawing more easy to understand... okay... but keep in mind that it's just straight up and down. So when we are finding the additional path length of ray two, we are going to be using the thickness here times two and we are not going to be doing some trigonometry to find the length of this hypotenuse or anything like that. Okay! So ray one is going to have some phase shift Φ 1 and then ray two will have some phase shift of Θ 2 and the total phase shift is going to be some integer plus a half times the wavelength in order to have destructive interference. So the phase shift of ray one is going to be half of a wavelength due to reflection from an interface where the second medium has a higher index of refraction than the first medium so this ray is coming through air, which has an index of refraction of 1.00 and then the second medium at this interface is magnesium fluoride, which has a higher index of refraction and so this reflection causes a phase shift of half a wavelength. And then ray two has a phase shift due to reflection here as well because it's going from a medium with index of refraction of 1.38 and then reflecting off an interface where the second medium has a higher index of refraction 1.52 and so this also has a λ over 2 phase shift as a result of that reflection but in addition to that there's a phase shift due to the extra path length that it travels and so we want to know how many wavelengths is this additional path length? And so that will be 2 times the thickness divided by the wavelength and that's the wavelength in the medium that it's traveling in so this will be the wavelength in magnesium fluoride. And so the wavelength in the anti-reflective coating is the wavelength in a vacuum or air because they are pretty much the same divided by the index of refraction of the magnesium fluoride. So this tells us the number of wavelengths— this fraction— and then we multiply that by the wavelength once it's back in the air. So we substitute n over λ in place of this λ n here because I am dividing by this λ n λ n is this fraction here and dividing by this fraction means multiplying by its reciprocal so I have that substituted here these λ's cancel and we are left with the phase shift for ray two of λ over 2 plus 2 times the thickness times the index of refraction of the magnesium fluoride. So I substitute this in for Φ 2 here and then add to that Φ 1 and this is the total phase shift. And now we are going to be solving for λ in a minute but first, we are going to add the λ over 2 plus λ over 2 which makes λ and then we'll subtract the λ from both sides and then we have this line here after we switch the sides around and then factor out the λ— the wavelength here— and we have λ times m plus a half minus 1 equals 2t times n and this is m minus a half and then divide both sides by that bracket and then we have the wavelength is 2 times the thickness times the index of refraction divided by m minus a half. So there are going to be multiple wavelengths that experience destructive interference and we want to find one that's in the visible range so we are going to just guess and check by trying different values of m; trying 0 will result in nonsense because we'll have a negative value for λ so never mind that one, if we put in 1 that will be a good first thing to try so put in value of 1 for m and so we have 2 times the thickness of 105 nanometers times 1.38—index of refraction of magnesium fluoride— divided by 1 minus a half and that works out to 580 nanometers and that is in the visible range, it's pretty much green— green is 550 nanometers so this is more or less the same as green— and that will be the color that is most strongly suppressed by this anti-reflection coating.
