OpenStax College Physics for AP® Courses, Chapter 27, Problem 27 (Problems & Exercises)

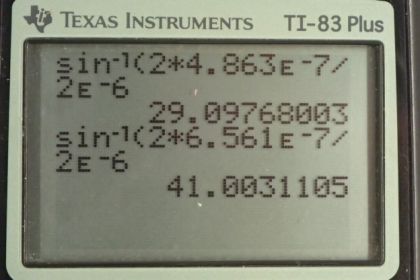
This is College Physics Answers with Shaun Dychko. We're told that the first order maximum for these four different wavelengths of light on a diffraction grating with 10,000 lines per centimeter is these four angles here. So each of these wavelengths gets one of these angles. And respectively. So the 4.099 times ten to the minus seven meters wavelength gets a first order maximum of 24.2 degrees given a diffraction grating of 10,000 lines per centimeter and so on. So we're going to calculate what the angles are to the first and second order Maxima with a diffraction grating having 5,000 lines per centimeter instead of 10,000. And we're going to compare our answers with what we have when we're dealing with the angles for 10,000 lines per centimeter. So given 5,000 lines per centimeter we need to know what is d, the separation between each line. And so we write the reciprocal of that 5,000 lines per centimeter and rewrite one centimeter for every 5,000 lines but every one centimeter has one times ten to the minus two meters in order to figure out the number of meters between each line which is two micrometers two times ten to the minus six meters. So here's the formula for the Maxima from a diffraction grating. That is the separation between the lines times sine of the angle to the maximum that equals the order of the maximum times the wavelengths of light. And we'll rearrange this to solve for theta by dividing both sides by d and then taking the inverse sine on both sides. So the angle is the inverse sine of the order times a wavelength divided by the separation between lines on the diffraction grating. So we're using this df here for each of these calculations and we have the angle for a wavelength a first order maximum, so a one, the first letter representing the wavelength that we're speaking about. And the second number representing the order that we're talking about. So this is the inverse sine of one which is the order, I didn't bother writing it, but I could, one times 4.099 times ten to the minus seven meters that's the wavelength and that's divided by the separation between slits in this new diffraction grating which is two times ten to the minus six meters giving a first order maximum at 11.8 degrees and so on and so on. We're calculating the first order maximum for each of the other wavelengths. So you see the denominator is the same each time, the order is the same it's one in each case and the wavelengths are these different wavelengths that I’ve written here and we get twelve and a half degrees and 14.1 degrees and 19.2 degrees. Now where things get interesting is when we calculate the second order maximum for each of these wavelengths with this new diffraction grating separation. And so we have the inverse sine of two because it's the second order so two which is the m here being multiplied by each of these wavelengths and then divided by two times ten to the six meters and taking the inverse sine of all that and we get 24.2 degrees, 25.7 degrees, 29.1 degrees and 41.0 degrees and all of these angles that we're getting for the second order Maxima are the same as angles we had for the first order Maxima with the original diffraction grating with 10,000 lines per centimeter and so in part c we can see that, we'll compare these lines per centimeter for each of these situations. So I have f for final, the final lines per centimeters is the number that we're using for calculations in this problem, the initial lines per centimeter is the lines per centimeter that we had given to us for these first order maximum angles and the final lines per centimeter is 5,000. The initial lines per centimeter is 10,000. And so these are different by a factor of a half. And so we can see that the initial lines per centimeter has been reduced by a factor of two. So we've divided by two and you reduce by a factor you're dividing by that number. So reducing by a factor of two. 5,000 is 10,000 reduced by a factor of two. And so the integral factor by which the lines per centimeters reduced which is two in this case that's the factor by which the lines per centimeters reduced from 10000 to 5000 is the order of the maximum which will have the same angle. And so we see that two is the order that we’ll have the same angle in this new diffraction grating. As for the first order maximum with the original diffraction grating. There we go.