Question
Make a drawing similar to Figure 23.14, but with the pendulum moving in the opposite direction. Then use Faraday’s law, Lenz’s law, and RHR-1 to show that magnetic force opposes motion.
<b>Figure 23.14</b> A more detailed look at the conducting plate passing between the poles of a magnet. As it enters and leaves the field, the change in flux produces an eddy current. Magnetic force on the current loop opposes the motion. There is no current and no magnetic drag when the plate is completely inside the uniform field.
Figure 23.14 A more detailed look at the conducting plate passing between the poles of a magnet. As it enters and leaves the field, the change in flux produces an eddy current. Magnetic force on the current loop opposes the motion. There is no current and no magnetic drag when the plate is completely inside the uniform field.
Question by OpenStax is licensed under CC BY 4.0
Final Answer

Please see the solution video.

Solution video

OpenStax College Physics for AP® Courses, Chapter 23, Problem 26 (Problems & Exercises)

OpenStax College Physics, Chapter 23, Problem 26 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right
Video Transcript
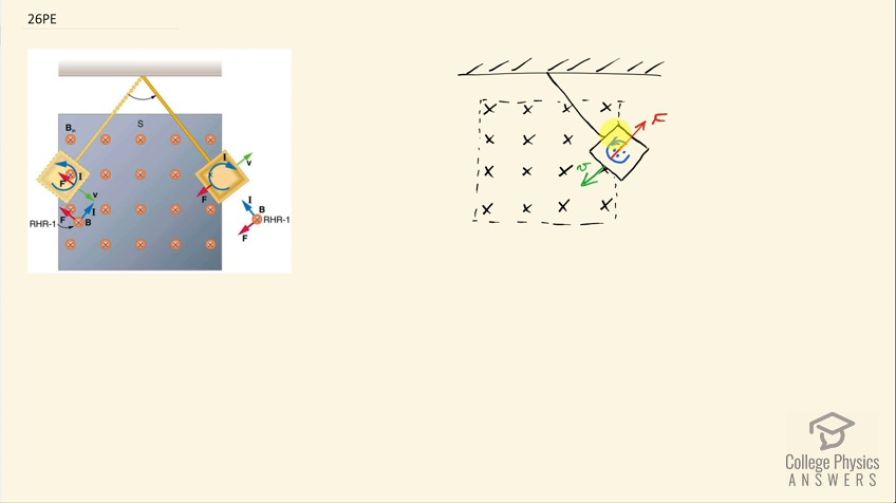
This is College Physics Answers with Shaun Dychko. This is a drawing that shows how magnetic damping occurs on a moving metallic square that's moving through a magnetic field or more specifically, it's entering a magnetic field. Once it's in the magnetic field fully, there's no magnetic damping on it anymore but when it's entering the field, there certainly is. So... this half of the square is in the magnetic field and the other half is not and so the magnetic flux through this square is increasing as this thing moves further into the field and that increase in flux will be opposed by the induced magnetic field created by the induced current. So because the flux is increasing, the direction of the induced magnetic field has to be in the opposite direction to the external field so the external field is going into the page and so the induced field has to come out of the page because this induced field attempts to stop this increase that's happening and that's Lenz's law basically that the direction of the induced magnetic field is going to oppose the external change in flux. So in order to have this magnetic field coming out of the page, we need to have a current that's going in this counter-clockwise direction and we can know that by grabbing the current with our right hand and have our fingers point out of the page and wherever you grab it, you will find that your thumb is pointing along this counter-clockwise direction. Okay! So now we have this induced current here. The next part is to figure out what force is being exerted on this current due to the external magnetic field? So to figure that out, let's grab this portion of current here our fingers go into the page because our many fingers go in the direction of the many magnetic field lines— that's a way to remember which part of your hand goes in the direction of the magnetic field lines— many fingers go into the page and then our thumb points in the direction of velocity of the moving charge and so that is this current and our thumb will be pointing this way and our palm is pushing in the direction of the force and that is this way opposite to the velocity. And if you were to grab just for... just anticipating a misunderstanding here if you were to grab this portion of the current, fingers into the page, thumb will be pointing this way your palm will be pointing with the direction of velocity but—there's a big BUT here— there's no magnetic field here externally magnetic field stops at this dotted black line and so that analysis is you can't do that, there is no force because there's no magnetic field there. Okay! So there's a force only on this portion of current that's in the magnetic field which is here. There we go! I suppose we are supposed to draw another picture over here and... but it would be the same idea and you would have the force going this way as the velocity is going this way. Okay! Good enough!