Question
What is the peak emf generated by rotating a 1000-turn, 20.0 cm diameter coil in the Earth’s magnetic
field, given the plane of the coil is originally perpendicular to the Earth’s field and is rotated to be parallel to the field in 10.0 ms?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 23, Problem 30 (Problems & Exercises)
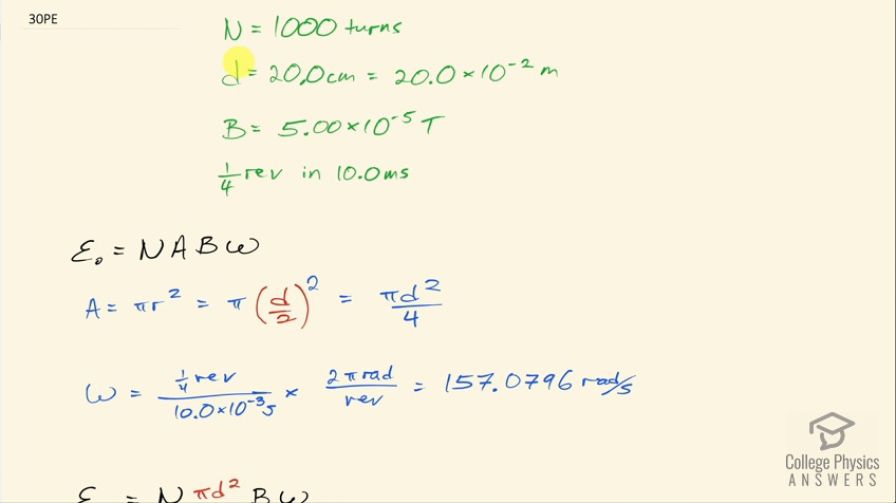
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We want to know the peak emf in a coil that has 1000 turns of wire, it's circular and so it has a diameter of 20.0 centimeters which is 20.0 times 10 to the minus 2 meters; it's in the Earth's magnetic field, which is 5.00 times 10 to the minus 5 tesla and it does one quarter of revolution in 10.0 milliseconds because we are told that with the Earth's magnetic field going one way, the plane of the coil begins perpendicular to that field and so the coil would be like this— it would be in the plane of the page— and then 10.0 milliseconds later, we have this same picture but the coil is now going to look like just this side-view. So here's dividing the picture... here's before and then after the turn so it starts like this and then it ends like this— this is one quarter of a turn— so that's why you get one quarter revolution and it takes 10.0 milliseconds to do that we are told so we use that information to figure out what the angular velocity is. So the peak voltage is the number of turns multiplied by its area and then multiplied by the magnetic field strength then multiplied by the angular velocity. So the area is π times radius squared we are given the diameter though so we substitute diameter divided by 2 in place of r and then we end up with π times d squared over 4 is the area. The angular velocity is one quarter revolution per 10.0 milliseconds written as 10.0 times 10 to the minus 3 seconds and then we convert this into radians per second by multiplying by 2π radians for every revolution and this is 157.0796 radians per second. I could have said initially that this is π over 2 radians of rotation but it's easier to think about it in terms of revolutions. Okay! So we have the peak voltage then is number of turns times π times diameter squared over 4 times magnetic field times angular velocity that's 1000 turns times π times 20.0 times 10 to the minus 2 meters squared over 4 times 5.00 times 10 to the minus 5 tesla— magnetic field strength— times 157.0796 radians per second and that is 0.247 volts.
