Question
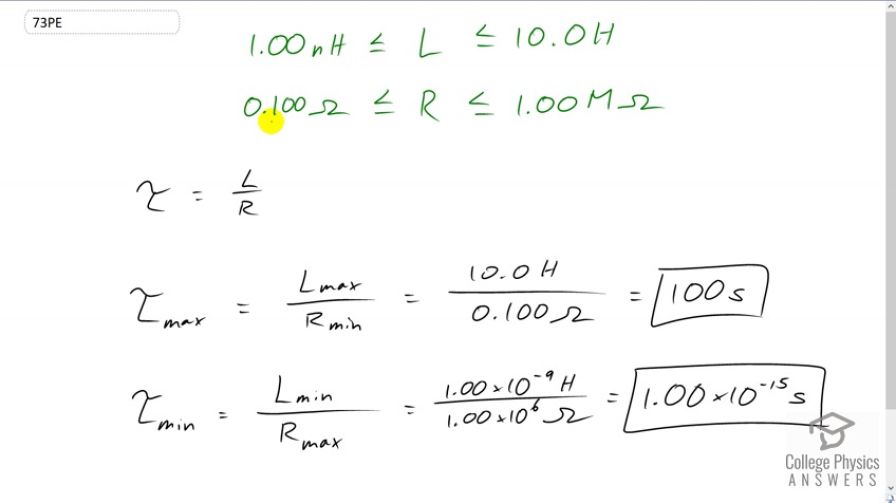
Suppose you have a supply of inductors ranging from 1.00 nH to 10.0 H, and resistors ranging from 0.100 Ω0.100 \textrm{ }\Omega to 1.00 MΩ1.00 \textrm{ M}\Omega. What is the range of characteristic RL time constants you can produce by connecting a single resistor to a single inductor?
Question by OpenStax is licensed under CC BY 4.0
Final Answer

τmax=100 s\tau_{max} = 100 \textrm{ s}

τmin=1.00×10−15 s\tau_{min} = 1.00 \times 10^{-15} \textrm{ s}

Solution video

OpenStax College Physics for AP® Courses, Chapter 23, Problem 73 (Problems & Exercises)

OpenStax College Physics, Chapter 23, Problem 73 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

Calculator Screenshots

  • OpenStax College Physics, Chapter 23, Problem 73 (PE) calculator screenshot 1
Video Transcript
This is College Physics Answers with Shaun Dychko. We have inductors ranging from a minimum of 1 nanohenry to a maximum of 10 henrys and some resistors ranging from 0.100 ohms upto 1 mega-ohms and we are asked to figure out what is the range of possible time constants we could make? So, the time constant is going to be the inductance divided by resistance. So to get the maximum time constant we want to maximize the numerator and minimize the denominator and so we will take maximum inductance divided by minimum resistance So that’s 10 henrys divided by 0.100 ohms which is 100 seconds will be the maximum time constant. And then for the minimum time constant, we want to minimize the numerator and maximize the denominator so we have 1 times 10 to the minus 9 henrys is the minimum inductance, that’s 1 nanohenry and we will divide that by 1 megaohm resistance which is 1 times 10 to the minus 15 seconds so that’s one femtosecond.