Question
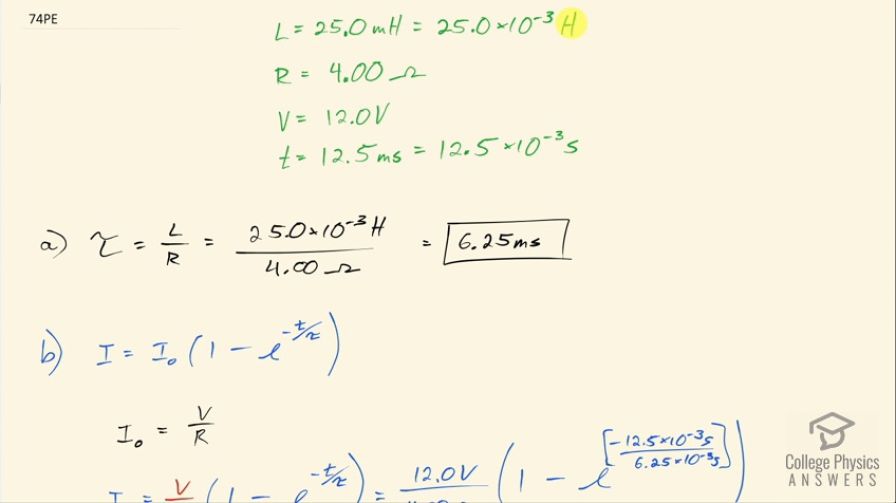
(a) What is the characteristic time constant of a 25.0 mH inductor that has a resistance of ? (b) If it is connected to a 12.0 V battery, what is the current after 12.5 ms?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 23, Problem 74 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a circuit with an inductor with inductance of 25.0 millihenries, which is 25.0 times 10 to the minus 3 henries and a resistance of 4.00 ohms and part (a) asks us for the characteristic time constant which is the inductance divided by the resistance. So that's 25.0 times 10 to the minus 3 henries divided by 4 ohms, which is 6.25 milliseconds. Part (b) says suppose this LR-circuit is connected to a 12.0 volt battery after 12.5 milliseconds, what will the current be? The current will be the peak current which is the current that's reached after a long time multiplied by 1 minus e to the power of negative time divided by this characteristic time constant. So the peak current is the voltage divided by the resistance this is the current that occurs after the battery has been connected for a really long time and there's no change in current anymore and therefore there's no induced emf in the inductor. So the induced emf in the inductor is the negative times the inductance multiplied by the rate of change of the current but after the battery is connected for a long time, the current is not changing so this factor becomes zero and there's no back emf due to the inductor in which case the current just flows through it—no problem— and the current will reach its peak of voltage divided by the resistance. So we substitute V over R in place of I naught and then plug in our other numbers to figure out what the current is after 12.5 milliseconds. So that's 12.0 volts divided by 4.00 ohms times 1 minus e to the power of negative 12.5 times 10 to the minus 3 seconds divided by this characteristic time constant of 6.25 times 10 to the minus 3 seconds and this gives 2.59 amps.
