Question
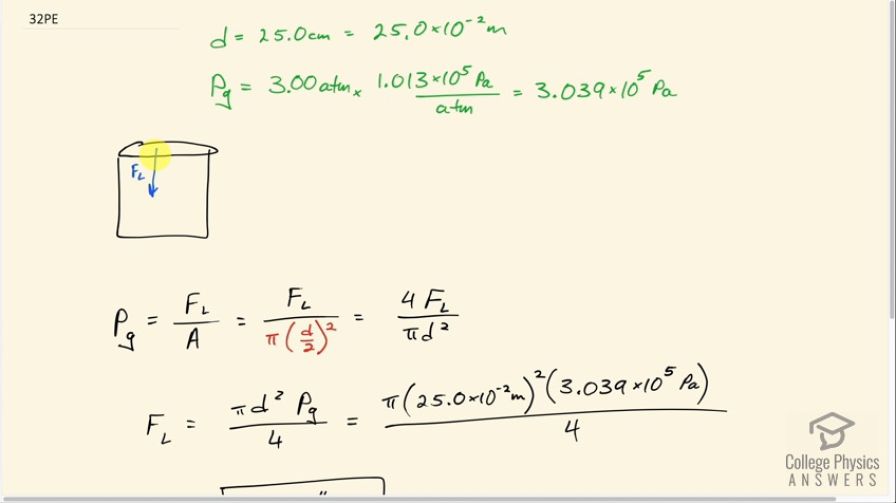
Pressure cookers have been around for more than 300 years, although their use has strongly declined in recent years (early models had a nasty habit of exploding). How much force must the latches holding the lid onto a pressure cooker be able to withstand if the circular lid is 25.0 cm in diameter and the gauge pressure inside is 3 atm? Neglect the weight
of the lid.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 11, Problem 32 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Given a pressure cooker with a lid diameter of 25.0 centimeters and a gauge pressure inside of 3.00 atmospheres, we want to know what force these latches holding the lid on need to exert downwards to keep the lid on the pressure cooker. Now we are given the gauge pressure which means we are given the pressure inside the pressure cooker the amount by which it exceeds atmospheric pressure. So there's some pressure inside that's going you know, resulting in a force upwards but that's being balanced by the atmosphere to some extent and this gauge pressure is just telling us what is not balanced by the atmospheric pressure and that's gonna be entirely compensated for by this force due to the latches. So the gauge pressure then is the force due to the latches divided by the area of the pressure cooker lid and at this stage here where we write down the things that we know, I have used that as a time to convert units so we don't have to worry about during our work here to do that. So the prefix 'centa' means times 10 to the minus 2 and 3.00 atmospheres multiplied by 1.013 times 10 to the 5 pascals per atmosphere is 3.039 times 10 to the 5 pascals. So we have force divided by area; the lid of the pressure cooker is circular and so its area is going to be π times radius squared and the radius is half the diameter. This works out to 4 times the latch force, F L, divided by π times the d squared and then we solve for F L by multiplying both sides by πd squared over 4. So the latch force then is π times 25.0 times 10 to the minus 2 meters squared times 3.039 times 10 to the 5 pascals divided by 4 which is 1.49 times 10 to the 4 newtons.
