Question
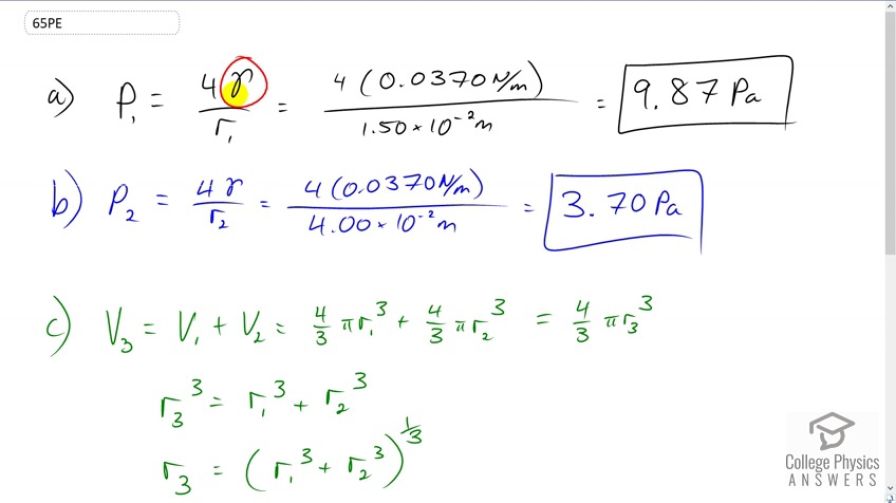
When two soap bubbles touch, the larger is inflated by the smaller until they form a single bubble. (a) What is the gauge pressure inside a soap bubble with a 1.50-cm radius? (b) Inside a 4.00-cm-radius soap bubble? (c) Inside the single bubble they form if no air is lost when they touch?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 11, Problem 65 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a formula for the gauge pressure inside a liquid sphere and it's four times the surface tension of the liquid divided by the radius of the sphere. So in the first case, we have four times gamma divided by radius one. This is the surface tension of soapy water which is 0.037 newtons per meter, times it by four and divide by the radius, 1.5 times ten to the minus two meters. This gives 9.87 Pascals. Now for the larger bubble in part B, we have four times gamma and gamma does not need a subscript because it's the same substance, same soapy water with the same surface tension, but we're dividing by r two now, the radius is now four times ten to the minus two meters. This gives 3.7 Pascals and as we expected, we have a lower pressure in a bigger bubble. Then in part C is what is the pressure in a bubble, we'll call it bubble three, consisting of the first two bubbles joined together? That'll mean that the volume of bubble three will be the sum of the volumes of bubbles one and two. That's four thirds pi r one cubed, that's the volume of the sphere, plus four thirds pi r two cubed. This is all going to equal four thirds pi r three cubed, the volume of the third new bubble. Now what we want to know is what is r three because that's the thing we need to substitute into our pressure formula. So we'll cancel the four thirds pi on both sides and we have r three cubed equals r one cubed plus r two cubed, and then we take the cube root of both sides or raise both sides to the exponent one third whichever way you like to think of it. So r three is one third power of r one cubed plus r two cubed. So the pressure in bubble three is four times gamma divided by r three. So that's four times gamma over r one cubed plus r two cubed all to the power of one third. That's four times 0.037 newtons per meter, divided by one and a half times ten to the minus two meters cubed, plus four times ten to the minus two meters cubed, all to the one third, giving us 3.64 Pascals. Sure enough that is even lower than that pressure, which we expected since this third bubble is even bigger.
