Question
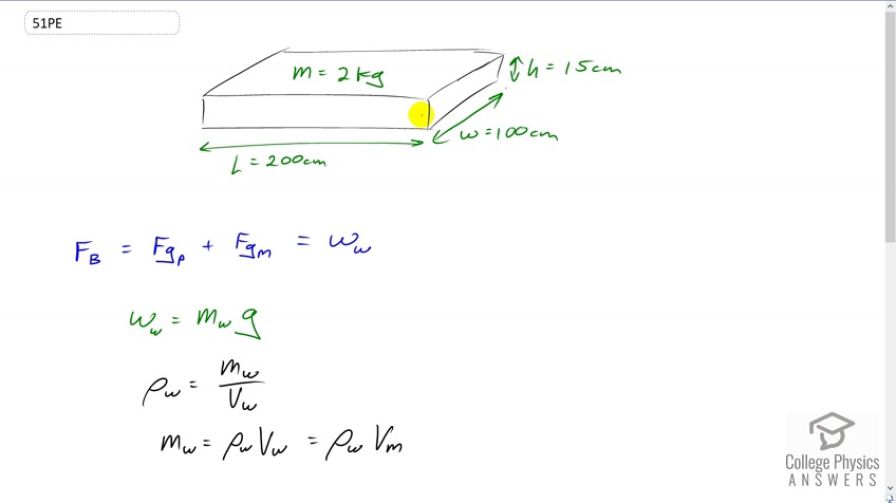
A twin-sized air mattress used for camping has dimensions of 100 cm by 200 cm by 15 cm when blown up. The weight of the mattress is 2 kg. How heavy a person could the air mattress hold if it is placed in freshwater?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 11, Problem 51 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This air mattress has dimensions of 200 centimeters by 100 by 15 centimeters thick and has a mass of two kilograms. The question is what is the maximum weight that this mattress can support? So that will happen when the mattress just begins to be completely submerged in which case the amount of water or the volume of water that it displaces will equal the volume of the mattress. So the buoyant force upwards provided on the mattress has to equal the force of gravity of the person that it's going to be supporting which will be downwards plus the force of gravity on the mattress itself. It's two kilogram mass there. This buoyant force is going to equal the weight of the water displaced by the mattress. The weight of the water displaced will be the mass of water displaced times g. We're going to express this mass in terms of the volume of the water because we know the volume of water displaced to be the volume of the mattress. So, we have density of water equals mass of the water divided by its volume and we can rearrange this to solve for mass by multiplying by V w on both sides. So mass of the water is density of water times volume of the water and now the volume of the water displaced will equal the volume of the mattress when the mattress just begins to be completely submerged at which point it'll be exerting its maximum possible buoyant force upwards. We will substitute into this weight of the water term here with density of water times the volume of the mattress which is the mass of the water displaced times g. Then we're going to solve for this term here. We want to know what is the greatest weight of a person that could be supported by the mattress so that means we'll subtract the force of gravity on the mattress itself on both sides to isolate force of gravity on the person on the left. So that's going to be density of water times the volume of the mattress which is its length times its width times height, times g minus mass of the mattress times g. We can factor the g out here. So we have 9.81 newtons per kilogram times one times ten to the three kilograms per cubic meter density of water, times the volume of the mattress converted into cubic meters by multiplying by one meter for every 100 centimeters, we do that three times, and then minus the two kilogram mass of the mattress. We get 2920 newtons is the maximum weight of the person that this mattress could support.
