Question
Scurrilous con artists have been known to represent gold- plated tungsten ingots as pure gold and sell them to the greedy at prices much below gold value but deservedly far above the cost of tungsten. With what accuracy must you be able to measure the mass of such an ingot in and out of water to tell that it is almost pure tungsten rather than pure gold?
Final Answer
Both in and out of the water, the mass measuring device needs milligram precision.
Solution video
OpenStax College Physics for AP® Courses, Chapter 11, Problem 50 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
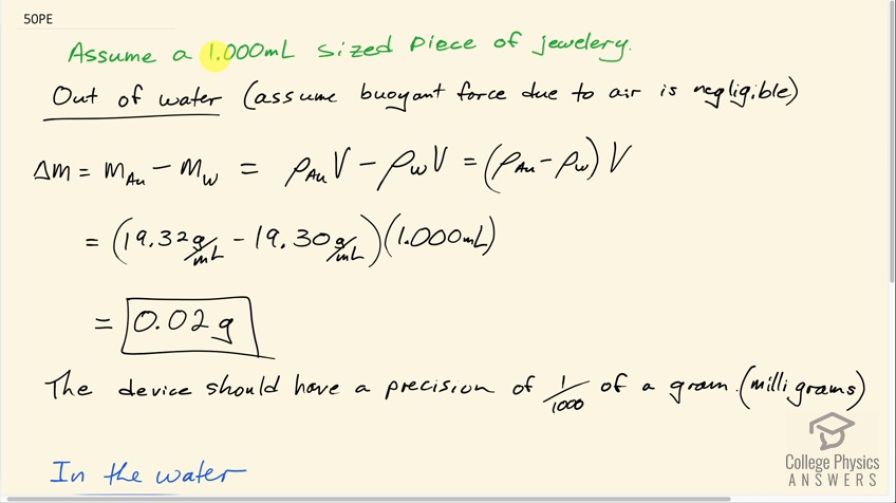
This is College Physics Answers with Shaun Dychko. In this question, we are going to see what precision a mass measuring device needs to distinguish between a piece of jewelry made out of gold versus a piece of jewelry made out of mostly tungsten and just plated with a negligible amount of gold. We'll assume that the jewelry has a volume of 1.000 milliliters; let's consider the case where it's out of the water and we'll find the difference in mass between the gold version versus the tungsten version and w is the chemical symbol for tungsten. So the mass of the gold then would be the density of gold multiplied by the volume that we have assumed here and then minus the density of tungsten minus the volume and this works out to 19.32 grams per milliliter—density for gold— minus 19.30 grams per milliliter— density for tungsten— times 1.000 milliliter which is 0.02 grams. So the device measuring the mass should have a precision to at least the next decimal point here which is the thousandths place which is the milligrams place. And I'm using the word 'precision' here; the question says 'accuracy' but accuracy means agreeing with other people's measurements whereas what they are really asking for is precision which is the number of decimal points that the device can output. Okay! If we are in the water then the mass difference will be the apparent mass of the gold versus the apparent mass of the tungsten and that is the mass of the gold minus the mass of the water displaced minus mass of the tungsten minus mass of the water displaced but the mass of water displaced will be the same in both cases because we are assuming these two samples each have the same volume of 1 milliliter and so these mass of the water terms cancel and we are left with just the same difference that we had out of the water, 0.02 grams, and so in the water the precision still needs to be milligrams.