Question
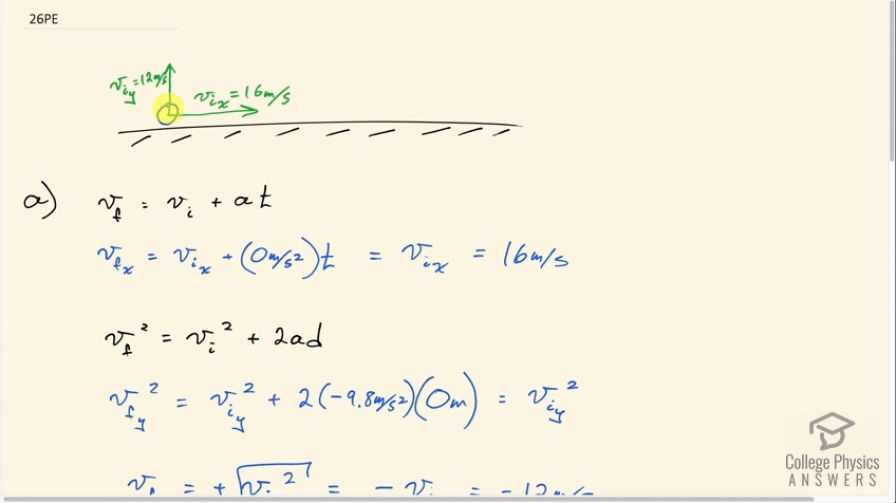
A ball is kicked with an initial velocity of 16 m/s in the horizontal direction and 12 m/s in the vertical direction. (a) At what speed does the ball hit the ground? (b) For how long does the ball remain in the air? (c)What maximum height is attained by the ball?
Final Answer
Solution video
OpenStax College Physics, Chapter 3, Problem 26 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
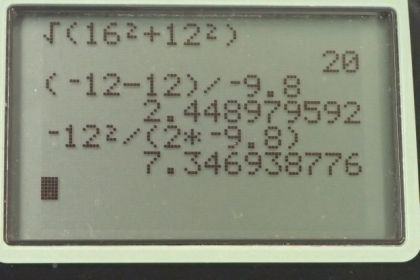
This is College Physics Answers with Shaun Dychko. A ball is kicked so that it has an initial x-component of its velocity of 16 meters per second and a y-component initially upwards of 12 meters per second. And part A asks us, at what speed does the ball hit the ground? So we need to find the resultant of its two components after it hits the ground over here somewhere. So we’ll deal with each component separately, we'll consider the x-component first. Then we're going to use this formula; we’ll say that the final velocity along any dimension be it x or y, equals initial velocity, plus acceleration, times time. But in the x-direction there's no acceleration because gravity acts only vertically, and so we put zero in for A. And so the time doesn't really matter and we end up with the initial x-velocity is the final x-velocity. In other words the x-component of the velocity of the ball doesn't change as it's moving. And so it's going to be 16 meters per second when it hits the ground. In the y-direction, we'll use this formula; which has the final velocity squared equals initial velocity squared, plus two times acceleration, times displacement. And we know the displacement is zero when it returns back to its initial height, this is the vertical displacement. When it hits the ground, vertical displacement is zero. So this entire term is zero and we're left with the final velocity in the y-direction squared, equals initial velocity in the y-direction squared. And so we take the square root of both sides. And when you do that, you don't know whether the left side is going to be the positive or the negative square root of the right side. So we can take our pick and we’ll choose negative because we can see from this, from our understanding of the physics here that the ball is going to be going in the downward direction like this. And so is going to have a negatively directed y-component of its velocity here and so we choose the negative. So the final y-velocity then is negative 12 meters per second. So the final velocity in total is going to be the Pythagorean sum of these two components. So we take the square root of the x-component squared, plus the y-component squared; so we have square root of 16 meters per second square, plus negative 12 meters per second squared. Giving us 20 meters per second, is the final velocity when the ball hits the ground. Part B asks, for how long does the ball remain in the air? We’ll use this formula to answer that question because we know everything in here except for t. We know the final y-velocity, the initial y-velocity and the acceleration. So we’ll subtract the initial y-component from both sides and then divide both sides by A. And we end up with this formula here after switching the sides around, so we have t equals the final velocity in the y-direction, minus the initial velocity in the y-direction, divided by the vertical acceleration. So that's negative 12 meters per second as the final y-velocity, that's what we discovered here. And then subtract from that the initial y-velocity which is positive 12 meters per second, and divide that by the acceleration, which is directed downwards at 9.8 meters second squared. And that gives 2.4 seconds as the amount of time it will spend in the air. Part C asks, what is the maximum height attained by the ball? So we need to understand that the y-velocity will be zero when the ball reaches its maximum height. And so knowing that, we can use this formula to figure out what that maximum height will be. So in the y-direction, we can say that the final velocity squared, which is zero, equals the initial y-velocity squared, plus two times vertical acceleration, times vertical displacement. So we'll solve for d by subtracting the initial y squared from both sides. And then divide both sides by 2a and we end up with the maximum height or the vertical displacement in other words, is negative the initial y squared over two, times acceleration. So that's negative of 12 meters per second squared, divided by two, times negative 9.8 meters per second squared, which is 7.3 meters maximum height.
Comments
very great! but I wish you'd slow down a bit
Hello infinity&eyond, thank you for the constructive feedback. I'm glad the solutions are helping as well. Admittedly I sometimes err on the side of "too efficient" (too fast in other words) as there are a lot of problems to solve. Have you noticed the playback speed controls in the video player? It's possible to slow down the playback speed. Maybe that would help?
All the best,
Shaun
thanks
In the question provided, for A why did we have to find the resultant Vector instead of just using the final vector of the vertical as the speed of the ball before it hits the ground?
Is it because the speed of the ball hitting the ground exists in both X and Y and not solely in Y?