Question
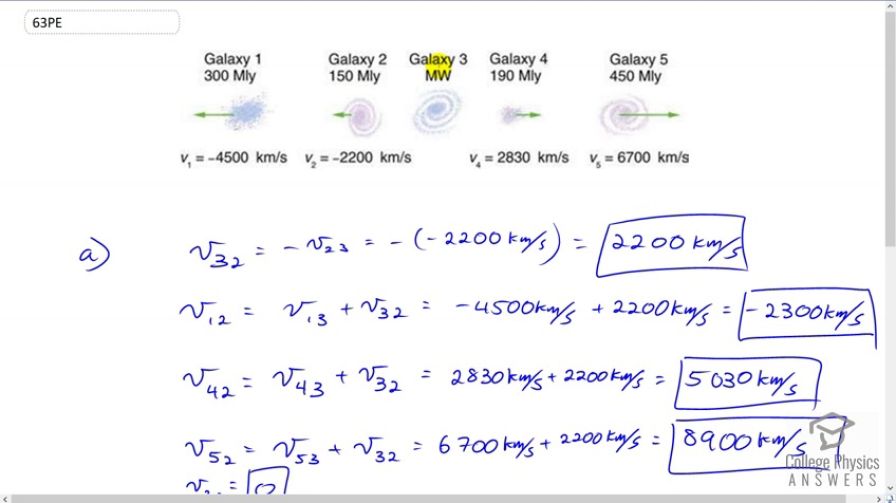
The great astronomer Edwin Hubble discovered that all distant galaxies are receding from our Milky Way Galaxy with velocities proportional to their distances. It appears to an observer on the Earth that we are at the center of an expanding universe. Figure 3.62 illustrates this for five galaxies lying along a straight line, with the Milky Way Galaxy at the center. Using the data from the figure, calculate the velocities: (a) relative to galaxy 2 and (b) relative to galaxy 5. The results mean that observers on all galaxies will see themselves at the center of the expanding universe, and they would likely be aware of relative velocities, concluding that it is not possible to locate the center of expansion with the given information.
<b>Figure 3.62:</b> Five galaxies on a straight line, showing their distances and velocities relative to the Milky Way (MW) Galaxy. The distances are in millions of light years (Mly), where a light year is the distance light travels in one year. The velocities are nearly proportional to the distances. The sizes of the galaxies are greatly exaggerated; an average galaxy is about 0.1 Mly across.
Figure 3.62: Five galaxies on a straight line, showing their distances and velocities relative to the Milky Way (MW) Galaxy. The distances are in millions of light years (Mly), where a light year is the distance light travels in one year. The velocities are nearly proportional to the distances. The sizes of the galaxies are greatly exaggerated; an average galaxy is about 0.1 Mly across.
Question by OpenStax is licensed under CC BY 4.0
Final Answer

a) v32=2200 km/sv_{32} = 2200 \textrm{ km/s}, v12=−2300 km/sv_{12} = -2300 \textrm{ km/s}, v42=5030 km/sv_{42} = 5030 \textrm{ km/s}, v52=8900 km/sv_{52} = 8900 \textrm{ km/s}, v55=0v_{55} = 0

b) v35=−6700 km/sv_{35} = -6700 \textrm{ km/s}, v15=−11200 km/sv_{15} = -11200 \textrm{ km/s}, v25=−8900 km/sv_{25} = -8900 \textrm{ km/s}, v45=−3870 km/sv_{45} = -3870 \textrm{ km/s}, v55=0v_{55} = 0

Solution video

OpenStax College Physics, Chapter 3, Problem 63 (Problems & Exercises)

OpenStax College Physics, Chapter 3, Problem 63 (PE) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right

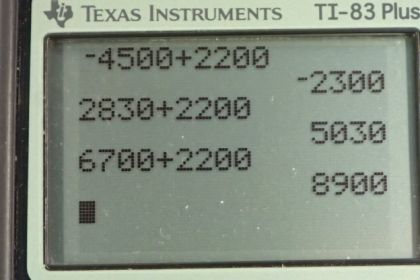
Calculator Screenshots

  • OpenStax College Physics, Chapter 3, Problem 63 (PE) calculator screenshot 1
  • OpenStax College Physics, Chapter 3, Problem 63 (PE) calculator screenshot 2
Video Transcript
This is College Physics Answers with Shaun Dychko. This picture gives us the velocity of four different galaxies with respect to an observer on the Milky Way which is our galaxy and we’re going to basically redraw this picture, giving velocities of all the galaxies with respect to an observer on Galaxy 2 in part a. And then again with respect to an observer on Galaxy 5 in part b. So our first step is to figure out what is the velocity of Galaxy 3 with respect to an observer on Galaxy 2, because this velocity is going to be used in all of our other relative velocity calculations since this picture gives us whole bunch of velocities with respect to Galaxy 3. So we need to know what the velocity of Galaxy 3 is with respect to 2 so we find that one first. It’s the negative of the velocity of Galaxy 2 with respect to 3, and so we’re given the velocity of Galaxy 2 with respect to 3. I mean you can put a little 3 after each of these subscripts here if you wanted, because they are all measured with respect to Galaxy 3. So that’s 2200 kilometers per second positive, and so that means from the perspective of somebody on Galaxy 2, Galaxy 3 appears to be moving to the right with this speed. Then we figure out what is the velocity of Galaxy 1 with respect to Galaxy 2, well it’s going to be the velocity of 1 with respect to 3 plus the velocity of 3 with respect to 2. We’ve written it this way so that the inner subscripts are the same and that’s why they cancel, leaving us with the outer subscripts, velocity 1 with respect to 2. So that’s negative 4500 kilometers per second plus 2200 kilometers per second that we’ve just calculated in the first part of this section a, and that gives negative 2300 kilometers per second is the velocity of Galaxy 1 according to an observer on Galaxy 2. Then Galaxy 4 with respect to 2 is velocity of 4 with respect to 3 plus 3 with respect to 2, the inner subscripts cancel giving us 42 there for subscripts and that’s 2830 kilometers per second plus 2200 kilometers per second, giving us 5030 kilometers per second is the velocity of Galaxy 4 with respect to 2. Then velocity 52 is velocity 53 plus velocity 32, and this is 6700 plus 2200 which is 8900. Just to be complete, I put the velocity of Galaxy 2 with respect to itself but that’s a bit silly because velocity of something with respect to itself is always zero because you can’t move with respect to yourself. So then we do all of this again, but from the perspective of somebody on Galaxy 5. So the first step is to figure out what is the velocity of the Milky Way Galaxy, Galaxy 3 with respect to 5. It’s the negative of the velocity of 5 with respect to 3, so that’s the negative of this, so negative 6700 kilometers per second. So somebody in Galaxy 5 will perceive Galaxy 3 as moving this way with this speed. Then the velocity of Galaxy 1 with respect to 5 is the velocity of 1 with respect to 3 plus the velocity of 3 with respect to 5, so that’s negative 4500 minus 6700, and that gives negative 11200 kilometers per second. v25 equals v23 plus v35, giving us negative 8900 kilometers per second. v45 is v43 plus v35, and notice the pattern you’re seeing everywhere here that these inner subscripts are cancelling, leaving us with the outer subscripts, and that’s always the way it is with relative velocities. That makes negative 3870 kilometers per second and of course the velocity of the Galaxy 5 with respect to itself is zero.