Question
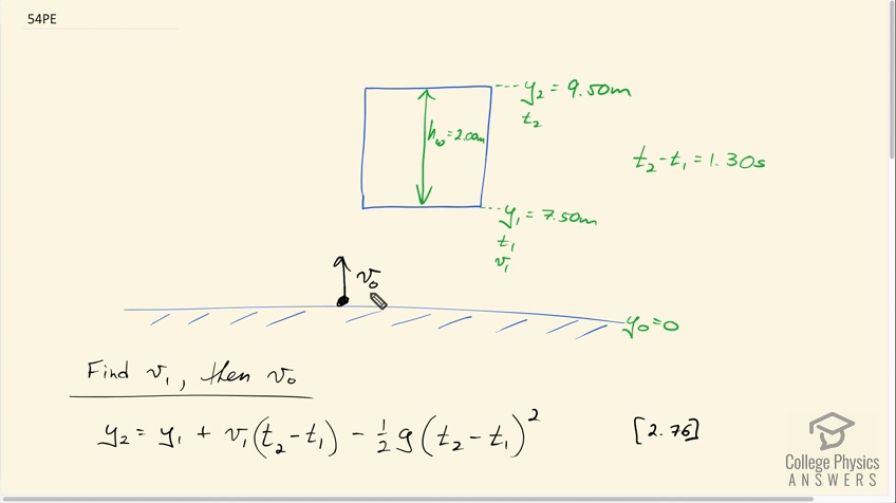
A ball is thrown straight up. It passes a 2.00-m-high window 7.50 m off the ground on its path up and takes 1.30 s to go past the window. What was the ball's initial velocity?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 2, Problem 54 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An object is thrown upwards with some initial speed v naught and the question here is to figure out what is this speed? And the information we are given is that when this object passes the bottom of a window, which is at a position 7.50 meters above the ground, it takes a further 1.3 seconds to get to the top of the window and the window height is 2 meters so that means this top position is 9.5 meters—that is 7.50 at the bottom plus the 2— and we are told that the time to get from the bottom of the window to the top is 1.3 seconds. So the time at the top of the window we'll label that with a subscript 2, t 2 and at the bottom of the window, we'll label that t 1. Then at the bottom of the window, there's also some velocity which we'll call v 1 that the ball is going at this position and our strategy to answer this question is to figure out what is the velocity at the bottom of the window going upwards, that's v 1, because we can figure that out in a fairly straightforward way using equation 76 but writing it this way and I'll explain that in a second. And then after we know what v 1 is then we can find v naught given this equation 77 here. Okay so one step at a time. Now the final position which is the top of the window, y 2, equals the initial position which we will take to be the bottom of the window and everything at the bottom of the window has a subscript 1 so it equals this initial position y 1, which is 7.50 meters, plus the speed at the bottom of the window, v 1, which is what we are going to try and find multiplied by the time it takes to get from the bottom to the top that is t 2 minus t 1 and that's a convenient difference because we know what that is— it's 1.30 seconds, the time it takes for the object to go from the bottom to the top of the window. And then minus one-half g times the time it takes to travel from the bottom to the top squared; that's equation 76. We are gonna solve this for v 1; first, we'll add this term to both sides and subtract this term from both sides and then switch the sides around so we have y 2 minus y 1 and then plus this one-half g times t 2 minus t 1 squared and then we divide both sides by t 2 minus t 1 and then we solve for v 1. So that's the top of the window minus the bottom of the window height plus one-half g times the time at the top of the window minus the time at the bottom of the window squared divided by that difference. So that's 9.50 meters minus 7.50 meters plus one-half times 9.80 meters per second squared— acceleration due to gravity— times 1.3 seconds— that's the time to get from the bottom of the window to the top—squared divided by 1.30 giving a speed of 7.90846 meters per second upwards at the bottom of the window. Then we use equation 77 which says that the final speed squared is the initial speed squared minus 2 times acceleration due to gravity multiplied by the displacement. So we are gonna solve this for v naught and we'll add 2g times y 1 minus y naught to both sides and then we get this line here after we switch the sides around as well then take the square root of both sides to get v naught. And so this is the square root of the velocity at the bottom of the window squared plus 2 times g times the position at the bottom of the window and the y naught I didn't repeat here because it is 0— we are taking this level here to be our reference level, 0— and this works out to square root of 7.90846 meters per second that we found before— that's v 1 squared— plus 2 times 9.80 meters per second squared times 7.50 meters—the height at the bottom of the window— square root all that and you get 14.5 meters per second was the initial velocity.
