Question
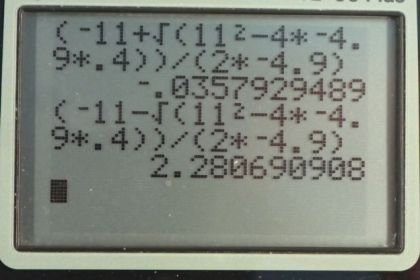
A very strong, but inept, shot putter puts the shot straight up vertically with an initial velocity of 11.0 m/s. How long does he have to get out of the way if the shot was released at a height of 2.20 m, and he is 1.80 m tall?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 2, Problem 48 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This shot putter launches a shot put with a velocity of 11.0 meters per second straight up and this is from a position of 2.20 meters that is y naught and sometime later, the shot put will eventually come back down and reach their head which is at a level of 1.80 meters. And so the question is how much time will it take for the shot put to go up and then back down to the level of the head? So equation [2.76] will be used to solve this. We have that the final position equals the initial position plus initial velocity times time minus one-half times acceleration due to gravity multiplied by time squared and we'll get everything on one side of the equation or the equal sign. So we'll have negative one-half gt squared plus v naught t plus y naught minus y equals 0. So we know everything in this equation except for the variable t and we can use the quadratic formula to solve this quadratic equation. Let's plug in some numbers; we have 9.8 meters per second squared in place of g 11.0 meters per second in place of v naught and then y naught is 2.20 meters— the initial position of the shot put— and then minus 1.80 meters which is the final position where the shot put would hit the person's head and then this simplifies to negative 4.90t squared plus 11t plus 0.40 equals 0. So the quadratic formula says that the time will be negative 11.0 meters per second plus or minus the square root of this coefficient of the linear term 11 squared minus 4 times the coefficient of the squared term— this negative 4.9— multiplied by the constant term 0.40 and all that gets divided by 2 times the coefficient of the squared term. This works out to negative 0.0358 seconds or 2.28 seconds and we'll take the positive answer because that's the one that has a physical interpretation that makes sense because it's gonna take some positive amount of time for the shot put to go up and then back down to the level of the head, 2.28 seconds.